The man sprang onstage dressed as a miner, complete with headlamp and pickaxe. After swinging the axe a few times, he proclaimed to the audience that he had discovered a “supermagnet”—a substance so strong it could attract even wood. A video screen above him appeared to prove him right: It showed wooden balls rolling up four ramps, seemingly unbound by gravity.
The man was not in fact a miner, but a mathematician—Kokichi Sugihara, of Meiji University in Tokyo, Japan. He was competing in the 2010 finals of the annual Best Illusion of the Year Contest in Naples, Florida. And, as the video went on to show, the balls were not really rolling uphill. A look at the back of the ramp structure showed that pillars that from the front had seemed vertical were in truth anything but, and that the ramps were pointing downhill, not uphill. The demonstration (which won that year’s top prize) was an illusion, and Sugihara had designed it together with an unusual collaborator: a computer program.
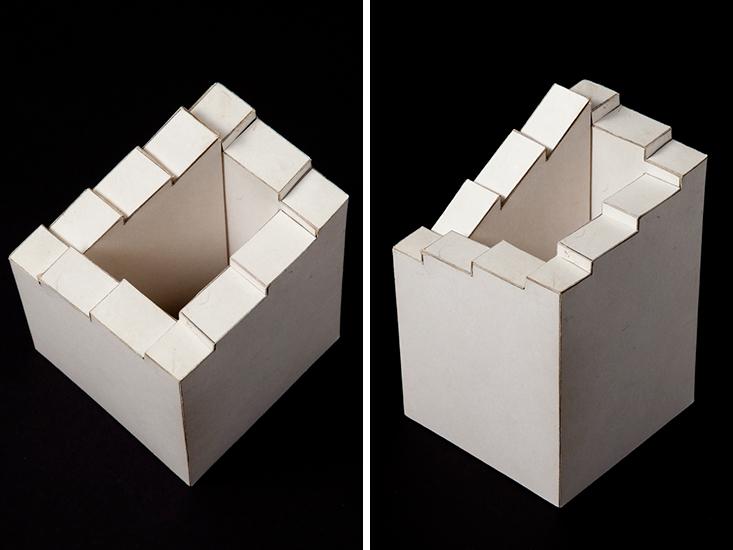
Sugihara didn’t originally set out to become a master illusionist. As a young mathematician in 1980, he was interested in robot vision and computer-assisted design. With those applications in mind, he created a computer program that could take a line drawing of a polygonal shape and come up with a list of three-dimensional objects that would create that drawing when projected onto a flat plane—akin to working backward from a shadow to the possible objects that could have cast it. To test his program, Sugihara fed it drawings of impossible-seeming objects, like M.C. Escher’s endless loop of stairs. He expected the program to reject the drawings, but to his surprise, in some cases it claimed to have found a solution: a three-dimensional object that looked just like the drawing. “I thought my software was incorrect,” Sugihara said.
On closer inspection, he realized that what was incorrect was his assumption that these “impossible” two-dimensional objects couldn’t exist in the three-dimensional world. He started making paper models of his program’s designs, and gradually, the robot and design applications fell by the wayside—his program wasn’t ideally suited to them, he discovered. Instead, he dedicated himself to exploring the strange constructions that came so easily to his program, and that appeared so baffling to human viewers. He had built an illusion machine.
Over the 34 years since then, Sugihara has used his illusion machine to create and build more than 100 illusions: impossible objects such as a solid model of Escher’s staircase, and intuition-defying motions such as balls rolling uphill. “Sugihara has come up with systematic ways of playing with what happens when the rules of the vision system fall apart,” said Arthur Shapiro, a neuroscientist at American University in Washington, D.C. Part scientist and part artist, his work is helping to elucidate the basic mathematics underlying how our brains construct our world.
The human brain routinely throws away many possible interpretations of the visual data it receives from the eyes. Given the brain’s limited resources and its need to interpret visual data quickly, it can’t afford to entertain every bizarre interpretation—it simply goes for the explanation that seems most likely, based on its past experiences and built-in visual processing machinery. For the most part—though not always—this explanation comes close enough to reality for all practical purposes, says Susana Martinez-Conde, a neuroscientist at SUNY Downstate Medical Center in Brooklyn, who runs the illusion contest with her colleague, Stephen Macknik. “It would be much more costly, from an evolutionary perspective, to be right 100 percent of the time.”
Sugihara believes that there’s a simple reason why his illusions appear so baffling: We are primed to perceive right angles, even when they do not exist. Many of the most compelling impossible pictures involve structures in which there are only three different directions of lines. When that happens, we seem to have an irresistible urge to perceive the three directions as mutually perpendicular wherever they meet.
Sugihara has tested this hypothesis by instructing his program to select the three-dimensional interpretation with the most right angles. Usually, he said, this makes the computer pick the same solid that humans perceive, supporting the hypothesis. When there is more than one solid with the maximal number of right angles, Sugihara suspects that the human visual system uses lighting cues to decide among them. The visual system tends to interpret brighter surfaces as facing upward and darker surfaces as facing downward, he says. Sugihara hopes eventually to build a module onto his program to test this guess.
Once we have made a right-angle interpretation, says Macknik, we tend to hang on to it even if it doesn’t make sense on a global scale. That’s because we process each bit of an image locally, leading us to “see” structures that are impossible. “We see the stairs in Escher’s staircase going up forever because locally, from one stair to the next, the angles are approximately correct,” he said. “There are enough small errors, which we don’t detect at the local level, to add up to a global impossibility.”
Sugihara’s ability to systematically manufacture illusions presents a new opportunity to probe our visual system. Another visual theory many psychologists entertain, Sugihara said, is that the vision system chooses the most symmetric possible interpretation of what it sees. He has begun to work with Akiyoshi Kitaoka, a psychologist who studies illusions at Ritsumeikan University in Kyoto, Japan, to figure out which of Sugihara’s illusions would best distinguish between this theory and the right-angle theory. The work should help researchers understand what priority we give to various visual shortcuts. As a rule, Martinez-Conde says, the lower down in the visual hierarchy an illusion gets processed, the harder it is to dispel. Depth illusions, which happen at a middle level of visual processing and describe much of Sugihara’s work, are “very resilient,” she says.
Sugihara’s prolific output relies not just on his program, but also on the impossible drawings that are its raw material. One tool he frequently uses to create these drawings is to move part of an object from the background of the picture to the foreground in a way that couldn’t physically happen. Another is to cut up a drawing and rearrange its parts so that the angles still seem perpendicular, but the new picture just doesn’t work in the real world. Using these and other simple tools, he has created a diverse gallery of impossible drawings. Most of these can’t be realized as three-dimensional objects, but Sugihara’s program has identified some that can.
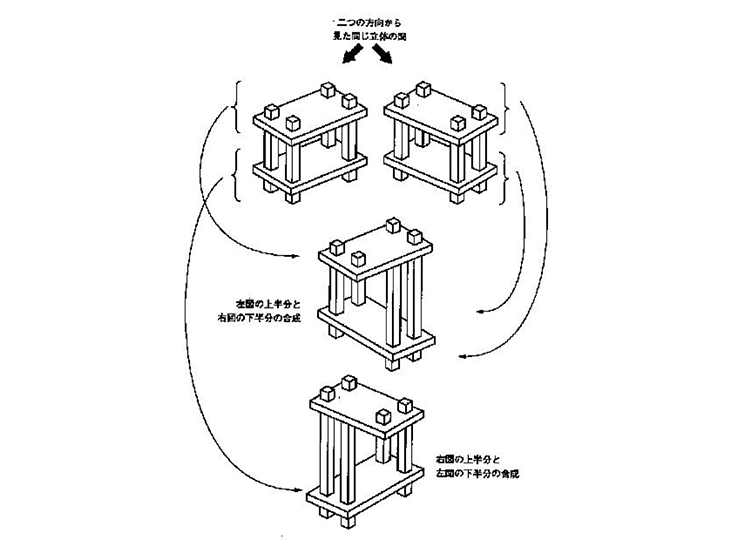
In recent years, he has added impossible motions to his repertoire—constructions in which the three-dimensional solid doesn’t look impossible, but objects move on it in ways that seem to defy the laws of physics. Sugihara’s program produces solids with straight lines and flat faces. Analyzing motion on such a solid is simple, and Sugihara has added a module to his program to do just that. To create the prize-winning illusion of balls rolling uphill, for example, Sugihara simply drew a sketch of the ramp apparatus, then instructed his program to come up with all the three-dimensional interpretations of the picture and identify the ones in which balls would roll “uphill.”
When we look at one of these impossible objects or motions, our cognitive realization that something is wrong isn’t strong enough to override the brain’s knee-jerk interpretation of the three-dimensional geometry. “Interpretation of images of three-dimensional solids can’t be controlled by the logical part of the brain,” Sugihara says.
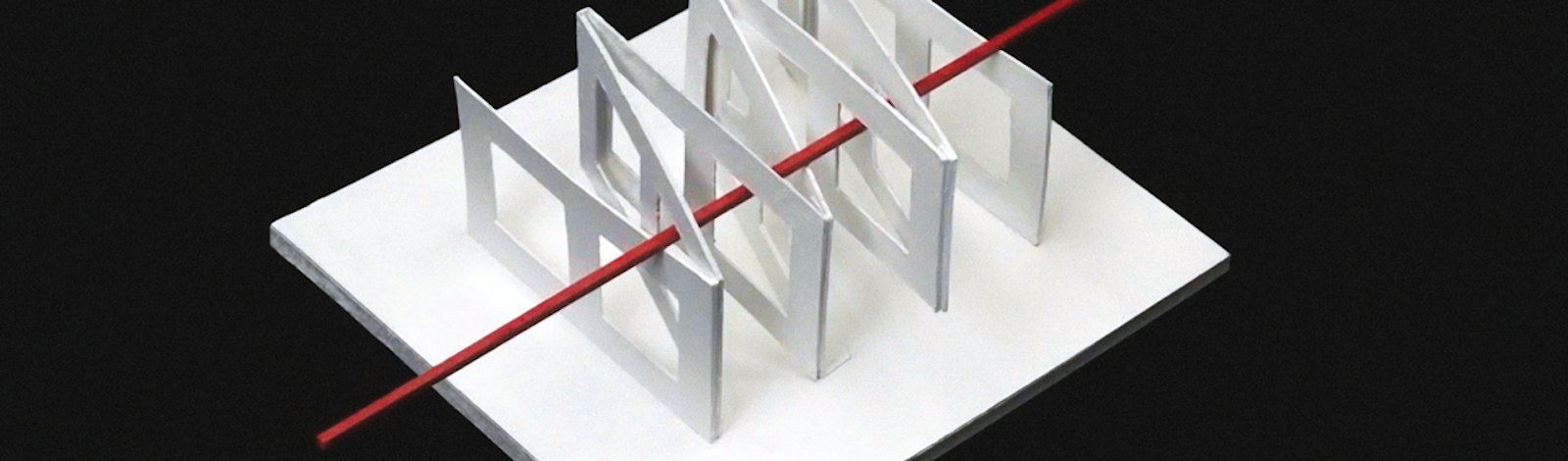
This phenomenon is readily apparent in Sugihara’s latest illusion, which he has agreed to unveil here. In it, a straight rod moves through the rungs of a folded ladder-like structure in a way that should be impossible if the rod can’t bend. To create the illusion, Sugihara sketched the ladder, then ran the drawing through his program and chose the three-dimensional interpretation that was most different from the natural human interpretation. Our right angle preference makes us visualize the top side of the ladder as flat, since that would make it perpendicular to all the support rungs. But the top isn’t flat: Several of the rungs come up high above the horizontal plane, enabling the rod to fit through them in ways that look impossible.
Sugihara’s program might give you the idea that sophisticated computer vision systems could be created that are free of our propensity to be hoodwinked. However, it’s unlikely that any computer program could see as well as humans without going through some sort of evolutionary process, said Dale Purves, a neuroscientist at Duke University in Durham, North Carolina. “Our vision has benefitted from millions or billions of years of evolution, depending on how you want to look at it,” he said. “I don’t think a machine will come close to working well until its connectivity is established by evolution, rather than the logic of engineers.”
And if robots do learn to see through evolution, they may inevitably be subject to the same illusions humans are. Beau Lotto, a neuroscientist at University College London, and David Corney, of Robert Gordon University in Aberdeen, Scotland, created robots that learned through trial and error to predict the shades of gray in synthetic nature scenes. Like humans, the robots were fooled by “White’s illusion,” in which identical shades of gray seem lighter or darker, depending on whether they are next to black or white stripes. It is also possible, though, that a computer vision system honed by evolution could be combined with programs such as Sugihara’s to offer the best of both worlds, Macknik says. “I have every confidence that there will eventually be things computer vision systems can do better than humans.”
For now, programs like Sugihara’s remain much less broadly effective than human vision. But they are unencumbered by our predilection to be fooled—something Sugihara plans to exploit to the fullest extent. After all, illusions aren’t just a professional interest for him, he says: “It’s my pleasure to make other people surprised.”
Erica Klarreich is a mathematics and science writer based in Berkeley, California. Her work has appeared in the 2010 and 2011 volumes of The Best Writing on Mathematics.