In the early 1800s, William Rowan Hamilton discovered a new kind of geometric space with nearly magical properties. It encoded motion and mathematics into a single, glinting geometric object.
This phenomenon birthed a field called symplectic geometry. Over the last few decades it has grown from a small collection of insights into a dynamic area of research with deep connections to more areas of math and physics than Hamilton ever could have imagined.
Symplectic geometry is ultimately the study of geometric spaces with a symplectic structure. But exactly what it means for a space to have a structure—let alone this particular structure—takes a little explaining.
Geometric spaces can be floppy like a tarp or rigid like a tent. “The tarp is very malleable but then you get, whatever, a bunch of sticks or scaffolding to shape it,” said Emmy Murphy of Northwestern University. “It makes it a more concrete thing.”
The least structured spaces are just collections of connected points (like the tarp). A line is a one-dimensional space of this sort. The surface of a ball is a two-dimensional version. The lack of structure in these spaces means it’s easy to deform them without fundamentally changing them: Wiggle the line and inflate, indent or twist the ball, and they’re both still the same in the eyes of topologists, who study these unstructured spaces.
“As far as topologists are concerned, if you start with the surface of a ball, you can stretch it any way you want, but as long as you don’t break it, it’s still the same space to them,” said Ailsa Keating of the University of Cambridge. “They are interested in the overall shape.”
Of course, when mathematicians talk about deforming a space, they don’t literally mean tugging at it with their hands. Instead, they transform spaces with functions: The coordinates of a point go into a function, and the coordinates for a new point come out. These transformations take every point of a space to a new point in the space. It’s the mathematical equivalent of shaking the tarp.
You can also add more structure to a space. This structure enhances the information the space contains, but also limits the ways you can deform it.
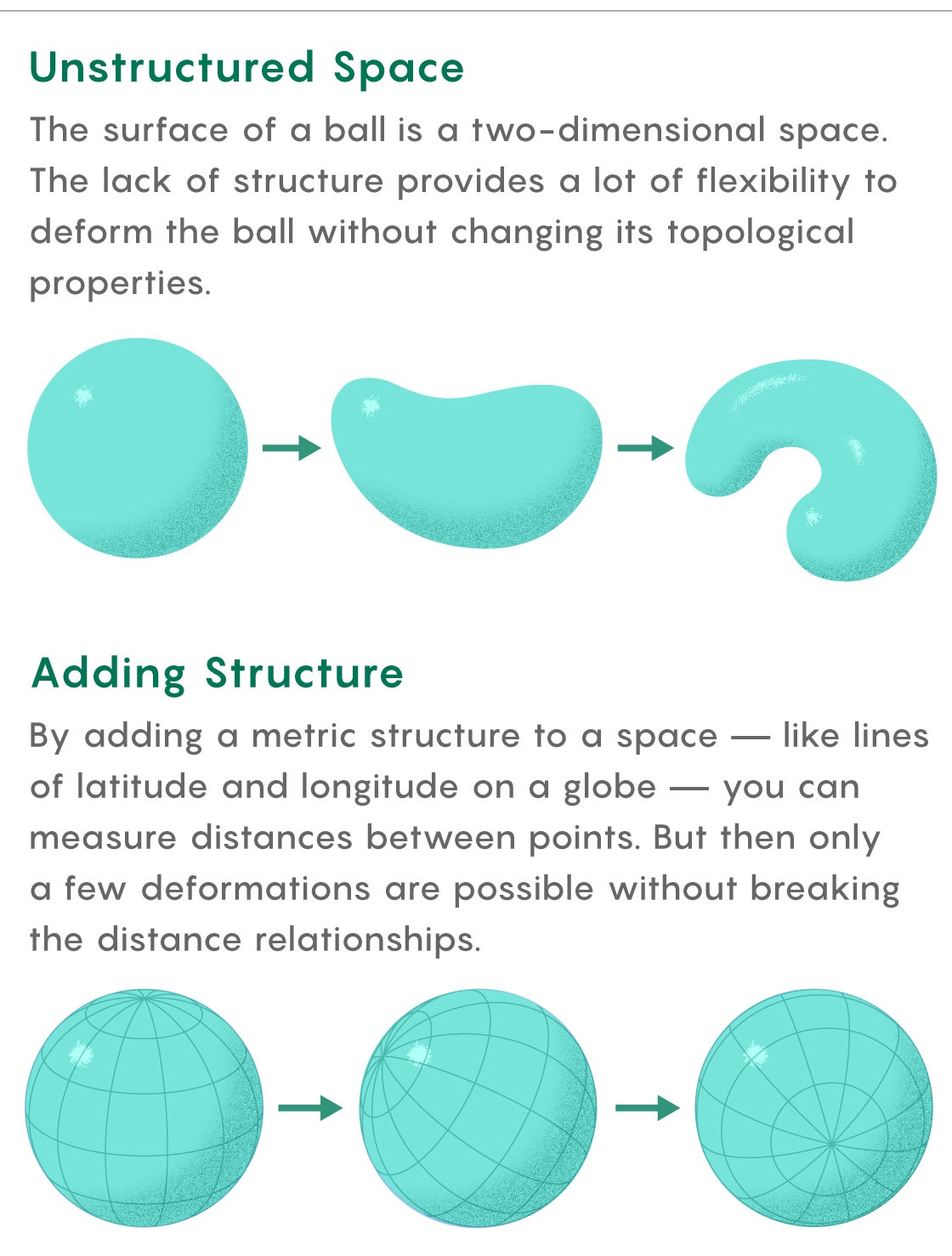
For example, you can add a metric structure to the surface of a ball, like longitude and latitude lines on a globe. This structure makes it possible to measure the distance between two points. But once that structure is in place, you can no longer inflate or indent the ball without breaking the original structure, because then you’re altering the distances between points. If you inflated the globe, for instance, New York and London would grow farther apart.
A symplectic structure is another structure you could add. It provides a way of measuring area in the space and allows you to change the space’s shape only if area measurements stay constant.
Hamilton discovered the first example of such a space while studying physical systems, like the motion of planets. As a planet moves through space, its position is defined by three coordinates that specify its location along the x, y and z axes. The points representing all possible positions of the planet form a three-dimensional space.
Hamilton observed that at each point in that three-dimensional space you can assign three additional coordinates that specify the planet’s momentum along each axis. Call them xm, ym and zm. Now you have six coordinates: three for position and three for momentum. These six coordinates define points in a new six-dimensional space.
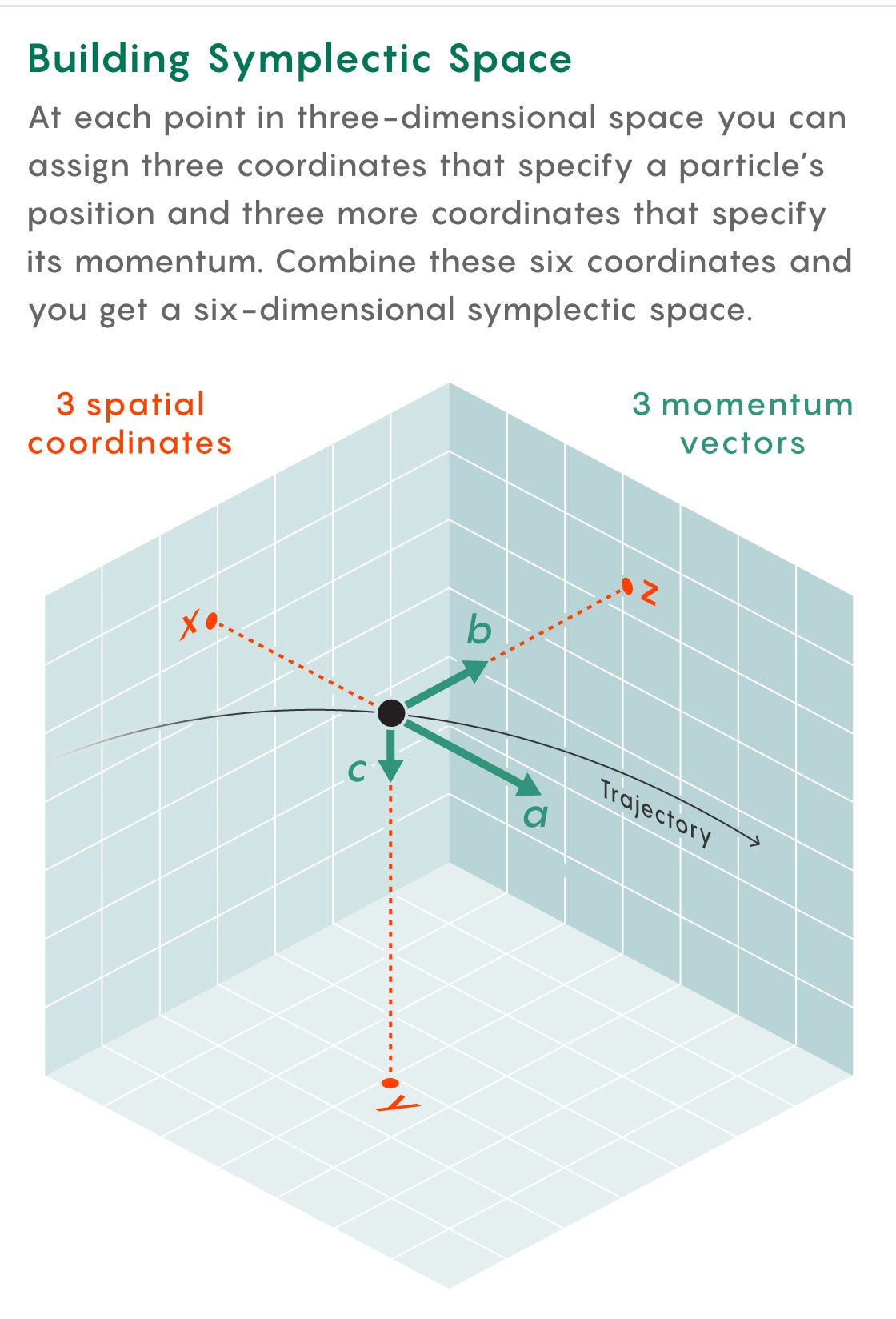
This six-dimensional space is an example of a space with a symplectic structure because it enables area measurements. Here’s how that works.
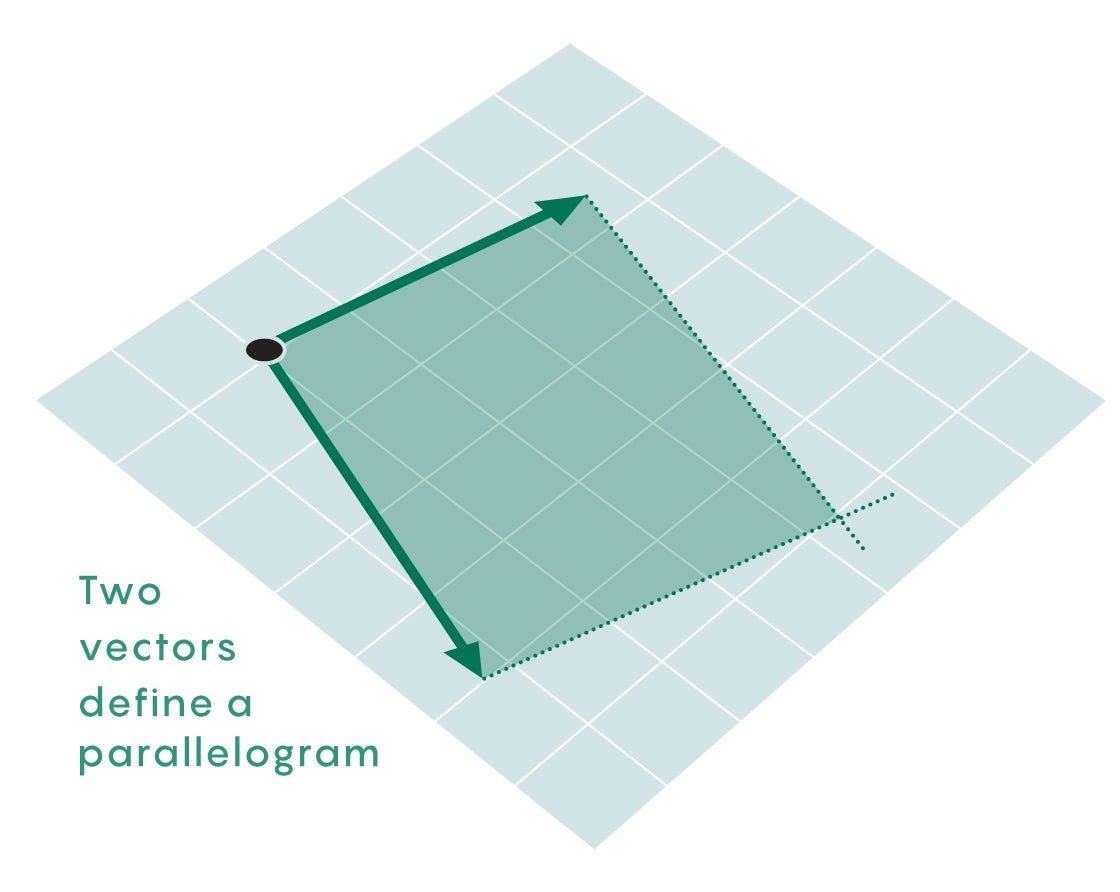
At each point in the space it’s possible to draw six “vectors,” or directed arrows, which correspond to the direction or momentum of the planet along the dimension in which the vector points. Because two vectors can define a parallelogram—a two-dimensional space with area—it’s possible to take two of the space’s vectors and measure an area.
But to ensure it’s a nonzero number, you have to pick specific pairs of vectors: those representing direction and momentum along the same axis. Mismatched vectors, like the z direction vector paired with the y momentum vector, form parallelograms with an area of zero.
These paired vectors also reflect another important property of symplectic spaces, their intrinsic connection to complex numbers. These numbers involve i, the square root of −1, and they take the form a + bi, where a is the real part and b is the imaginary part. One way of defining six-dimensional symplectic space is with three complex numbers, with each number’s two parts supplying two of the coordinates. Those two parts also correspond to the two vectors that we pair to measure area.
So for each point, the x-based direction and momentum vectors (for example) not only provide a way to measure area, but also make up one of the three complex numbers that define the space. This relationship is reflected in the name symplectic, which derives from the Greek word sumplektikós, the equivalent of the Latin-based “complex,” both of which mean “braided together”—evoking the way the symplectic structure and the complex numbers are intertwined.
It’s also one of the main reasons symplectic spaces captured mathematicians’ imaginations. “Mathematicians were already interested in complex numbers; they were already interested in the motion of planets,” Murphy said. “So if you come along and say, ‘There is this geometry that shows why these two things are different manifestations of the same underlying structure,’ of course math will run with it.”
Symplectic geometry studies transformations of spaces that preserve the symplectic structure, keeping area measurements constant. This allows for some freedom, but not too much, in the types of transformations you can employ. As a result, symplectic geometry occupies a kind of middle ground between the floppy topology of a tarp and the rigid geometry of a tent. The types of transformations that maintain the symplectic structure are called Hamiltonian diffeomorphisms, after the discoverer of the phenomenon.
But while Hamilton discovered the first example of a symplectic space, there was no reason it had to end there. After a while, mathematicians began to think about what symplectic phenomena would look like in geometric spaces unrelated to the physical world.
“Mathematicians are always all about generalizing, so maybe we want to say, ‘What would classical mechanics look like if, instead of living in three-dimensional space, we lived in eight-dimensional space?’” Murphy said.

Beginning in the 1960s, Vladimir Arnold made several influential conjectures that captured the specific ways in which symplectic spaces are more rigid than ordinary topological ones (like the floppy sphere). One of them, known as the Arnold conjecture, predicts that Hamiltonian diffeomorphisms have a surprisingly large number of “fixed” points, which don’t move during a transformation. By studying them, you can put your hands on just what it is that makes a symplectic space different from other kinds of geometric spaces.
In the late 1980s, a mathematician named Andreas Floer developed a theory called Floer homology, a powerful framework that is now the primary way mathematicians investigate symplectic phenomena. It uses objects called pseudoholomorphic curves, which, in a roundabout way, allow mathematicians to count fixed points and establish that a certain minimum number of them are intrinsic to the symplectic space.
“[Floer homology] allows you to prove that you can’t just shake the fixed points away,” said Keating. “It’s what allows you to prove that the fixed points have to be there.”
As the theory of symplectic geometry has grown, it has found connections with an increasingly wide range of topics in mathematics and physics, from string theory to low-dimensional topology to the study of a bewildering mathematical duality called mirror symmetry. In just one recent example, symplectic geometry proved instrumental in solving a problem from topology called the rectangular peg problem, as Quanta reported in “New Geometric Perspective Cracks Old Problem About Rectangles.”
Yet for many mathematicians, the appeal of symplectic geometry has little to do with the ways it relates to physics or other areas of math. It lies in the marvel that it exists at all.
“We start finding beauty in the structure itself, regardless of how it connects to anything else,” Murphy said.
Correction: July 29, 2020
A graphic in the original version of the story showed the trajectory of a particle that was inconsistent with the momentum vectors in the graphic. It has been revised accordingly.
Lead image: Olena Shmahalo/Quanta Magazine