In 1974, Roger Penrose, a British mathematician, created a revolutionary set of tiles that could be used to cover an infinite plane in a pattern that never repeats. In 1982, Daniel Shechtman, an Israeli crystallographer, discovered a metallic alloy whose atoms were organized unlike anything ever observed in materials science. Penrose garnered public renown on a scale rarely seen in mathematics. Shechtman won the Nobel Prize. Both scientists defied human intuition and changed our basic understanding of nature’s design, revealing how infinite variation could emerge within a highly ordered environment.
At the heart of their breakthroughs is “forbidden symmetry,” so-called because it flies in the face of a deeply ingrained association between symmetry and repetition. Symmetry is based on axes of reflection—whatever appears on one side of a line is duplicated on the other. In math, that relationship is reflected in tiling patterns. Symmetrical shapes such as rectangles and triangles can cover a plane with neither gap nor overlap, and in an ever-repeating pattern. Repeated patterns are called “periodic” and are said to have “translational symmetry.” If you move a pattern from place to place, it looks the same.
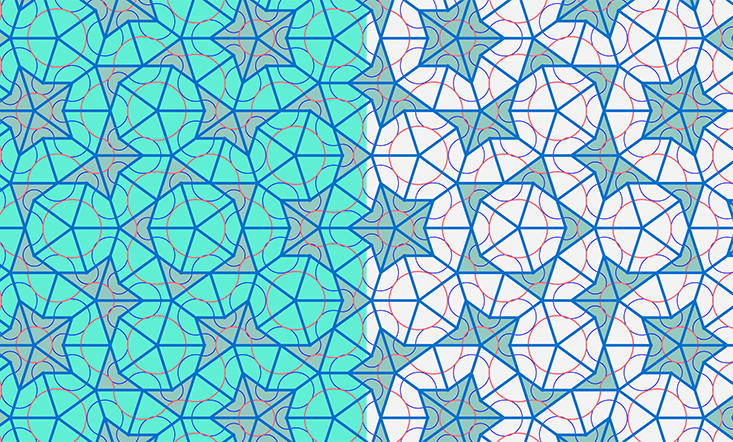
Penrose, a bold, ambitious scientist, was interested less in identical patterns and repetition, and more in infinite variation. To be precise, he was interested in “aperiodic” tiling, or sets of tiles that can cover an infinite plane with neither gap nor overlap, without the tiling pattern ever repeating itself. That was a challenge because he couldn’t use tiles with two, three, four, or six axes of symmetry—rectangles, triangles, squares, and hexagons—because on an infinite plane they would result in periodic or repeated patterns. That meant he had to rely on shapes believed to leave gaps in the tiling of a plane—those with forbidden symmetries.
Penrose turned to five-axis symmetry, the pentagon, to create his plane of non-repeating patterns, in part, he has said, because pentagons “are just nice to look at.” What was remarkable about Penrose tiles was that even though he derived his tiles from the lines and angles of pentagons, his shapes left no awkward gaps. They snugged together perfectly, twisting and turning across the plane, always coming close to repetition, but never quite getting there.
It was as if Penrose wrote a work of fiction about a new animal species, only to have a zoologist discover that very species living on Earth.
Penrose tiling captured public attention for two major reasons. First, he found a way to generate infinitely changing patterns using just two types of tiles. Second, and even more spectacular, his tiles were simple, symmetrical shapes that on their own betrayed no sign of their unusual properties.
Penrose made several versions of his aperiodic tile sets. One of his most famous is known as the “kite” and the “dart.” The kite looks like the kids’ toy of the same name, and the dart looks like a simplified outline of a stealth bomber. Both divide cleanly along axes of symmetry and each has two simple, symmetrical arcs on their surface. Penrose established one placing rule: for a “legal” tile placement these arcs must match up, creating contiguous curves. Without this rule, kites and darts can be placed together in repeating patterns. With this rule, repetition never comes. The kite and the dart tile forever, dancing around their five axes, creating starbursts and decagons, winding curves, butterflies and flowers. Shapes recur but new variations keep creeping in.
Edmund Harriss, an assistant clinical professor in mathematical studies at the University of Arkansas, who wrote his Ph.D. thesis on Penrose tiles, offers a comparison. “Imagine you’re on a world that is just made up of squares,” Harriss says. “You start walking, and when you get to the edge of the square, and the next square is exactly the same, you know what you’re going to see if you walk forever.” Penrose tiling has the exact opposite nature. “No matter how much information you have, how much you’ve seen of the tiling, you’ll never be able to predict what happens next. It will be something that you’ve never seen before.”
One of the curious aspects of aperiodic division of the plane is that information about positioning is somehow communicated across great distances—a Penrose tile placed in one position prevents the placement of other pieces hundreds (and thousands and millions) of tiles away. “Somehow a local constraint imposes a global constraint,” says Harriss. “You impose that at no scale will these tiles give you something that is periodic.” You can be faced with the choice of placing, say, a kite in one area, or a dart in a far distant location. Either tile will work, but not both.
The tiles, which form an infinite non-repeating pattern, express the Fibonacci ratio, also known as “the golden ratio.” Two numbers are said to have the golden ratio if the ratio of the smaller number to the larger is the same as the ratio of the larger number to the sum of the two numbers. In this case, the ratio of the area of the kite to that of the dart is the golden ratio. The ratio of the longer side of the kite to its shorter side is also the golden ratio.
Penrose tiles can also be subdivided into smaller versions of themselves. A kite comprises two smaller kites and two halves of a dart. A dart comprises a smaller kite and two halves of a dart. (In any valid Penrose tiling, all those half-darts line up with one another. Mathematically, this allows them to be treated as whole darts.) “Suppose I have a patch of Penrose tiling, and that has A kites and B darts,” says Harriss. “If I subdivide, I’m going to have 2A+B kites, and A+B darts.”
If you do this kind of substitution an infinite number of times, you can work out the overall ratio of each type of tile, were they laid out on a never-ending plane. In such calculations, a repeating pattern always results in a rational ratio. If the ratio is an irrational number, it means that the pattern will never fully repeat. Not only do Penrose tiles yield an irrational number, their relationship is the Fibonacci ratio—the ratio of darts to kites is identical to the ratio of kites to the total number of tiles.
Given that Fibonacci seems to appear everywhere in nature—from pineapples to rabbit populations—it was all the more odd that the ratio was fundamental to a tiling system that appeared to have nothing to do with the physical world. Penrose had created a mathematical novelty, something intriguing precisely because it didn’t seem to work the way nature does. It was as if he wrote a work of fiction about a new animal species, only to have a zoologist discover that very species living on Earth. In fact, Penrose tiles bridged the golden ratio, the math we invent, and the math in the world around us.
Penrose could not have known when he embraced forbidden symmetry that he was part of a mental shift that would lead to a new field of materials science. After all, symmetry is fundamental to both pure mathematics and the natural world. Astrophysicist Mario Livio has described symmetry as “one of the most essential tools in deciphering nature’s design.” Nature uses squares and hexagons for the same reasons human designers do: They are simple, efficient, and orderly. If pentagons seemed impractical for something as simple as a floor-tile pattern in interior design, they were thought impossible for the formation of atoms in solid materials like crystals.
Crystals are made up of three-dimensional lattices of atoms. Crystals grow by adding more atoms and extending the lattices. This happens most efficiently when atoms line up in repeating patterns. For decades, the story ended there: Crystals were repeating structures. The end.
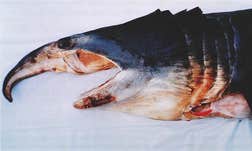
Then, in 1982, Shechtman was on sabbatical from Haifa’s Technion University at the National Bureau of Standards. He was mucking about in the lab with an aluminum-manganese alloy. The diffraction patterns created by his crystalline structures didn’t seem to reflect any of the standard symmetries known to crystallographers. In fact, the atoms appeared to be arranged in the very pentagons, rhombi, kites, and darts that Penrose had unleashed upon the math world.
“I was familiar with Penrose tiles, of course,” Shechtman says. But he had no reason to suspect they related to his alloy. “I didn’t know what it was. I repeated my experiments time and again over the next months. At the end of my sabbatical, I knew exactly what it was not, but I still didn’t know what it was.”
To understand what he had found, Shechtman had to defy the same intuition and conventional wisdom as Penrose. He had to embrace forbidden symmetry with its pentagonal confusion and non-repetition. Back in Israel, he came to the unwilling conclusion that he had found a non-repeating crystalline atomic structure. Though neither he nor anyone in the materials science world could at first bear to call his discovery a crystal. They called them “quasicrystals” instead.
Quasicrystals have low friction and wear, which makes them ideal coatings for razors and surgical instruments.
It was as though Penrose’s fanciful mathematics had forced itself into the natural world. “For 80 years, a crystal was defined as ‘ordered and periodic,’ because all crystals studied from 1912 on were periodic,” Shechtman says. “It wasn’t until 1992 that the International Union of Crystallography established a committee to redefine ‘crystal.’ That new definition is a paradigm shift for crystallography.”
It was more than mere mental inertia that made it so hard to understand and absorb Shechtman’s discovery. Aperiodic crystalline structures weren’t just unfamiliar; they were supposed to be unnatural. Remember that the placement of one Penrose tile can affect things thousands of tiles away—local constraints create global constraints. But if a crystal forms atom by atom, there should be no natural law that would allow for the kind of restrictions inherent to Penrose tiles.
It turns out crystals don’t always form atom-by-atom. “In very complex intermetallic compounds, the units are huge. It’s not local,” says Shechtman. When large chunks of crystal form at once, rather than through gradual atom accretion, atoms that are far apart can affect one another’s position, exactly as do Penrose tiles.
As with many taboos, forbidden symmetry ultimately gained acceptance as a valid form of natural existence. Not only did quasicrystals become the subject of a new field of academic research, they turned out to have many useful properties that result from their unusual structure. Their irregular atomic configuration, for instance, gives them a low surface energy, which means that not much sticks to them. As a result, quasicrystal coatings have found their way into nonstick cookware. (When Penrose created his novel tiles, there was no reason to think it would have a bearing on crystallography, let alone on frying an egg.) Quasicrystals also tend to have low friction and wear, which makes them ideal coatings for razors and surgical instruments, or any sharp tool that touches the human body.
Because the structures of quasicrystals never repeat, they create unique diffraction patterns out of electromagnetic radiation. Photonics researchers are interested in how they influence optical transmission, reflectivity, and photoluminescence. Supercool them, and their electrical resistance soars to near-infinite levels. But they also absorb infrared radiation, getting very hot, very quickly. That makes them a much-valued additive to 3D printers, which often use plastic powder as a source material. When a quasiperiodic powder is mixed in, Shechtman explains, and beamed with an infrared light, the quasiperiodic powder “heats up extremely quickly and melts the surrounding plastic particles, which stick together.”
Nobody knows how the story of forbidden symmetry ends. Mathematicians continue to explore the properties of Penrose tiles. Quasicrystals remain the subject of both basic and applied research. But it has been an incredible journey so far. In the past 40 years, five-axis symmetry has gone from impractical to valuable, from unnatural to perfectly natural, from forbidden to mainstream. It’s a transformation for which we can thank two scientists who pushed past conventional wisdom to uncover a remarkable new form of infinite variation in nature.
Patchen Barss is a Toronto-based journalist and author. He is currently working on a book about the relationship between pure mathematics and the natural world.
The lead image was constructed from two images, which were originally found on AMES lab/Wikimedia Commons and Parcly Taxel/Wikimedia Commons.