Gravity Waves and Neutrinos

Explore

How does the physics community deal with the subsequent work of a scientist whose earlier work has been regarded as incorrect? An interesting case of this involves Joseph Weber whose claim to have observed gravitational waves was rejected by virtually all of the physics community, although Weber himself continued to defend his work until his death in 2000. In the course of this defense Weber made a startling suggestion regarding the scattering of neutrinos. I will summarize the history of gravity waves including the rejection of Weber’s claim around 1975, his later work on gravity waves, and examine the reaction of the physics community to his neutrino hypothesis.

In 1942, the distinguished sociologist of science, Robert Merton, described universalism as one of the norms of science.¹ “Universalism,” he remarked, “finds immediate expression in the canon that truth-claims, whatever their source, are to be subjected to pre-established impersonal criteria: consonant with observation and with previously confirmed knowledge. The acceptance or rejection of claims entering the lists of science is not to depend on the personal or social attributes of their protagonist; his race, nationality, religion, class, and personal qualities are as such irrelevant. … The Haber process cannot be invalidated by a Nuremberg decree nor can an Anglophobe repeal the law of gravitation” (Merton 1942, p. 118). Merton recognized that universalism was an ideal that was not always practiced. He believed, however, that most scientists subscribed to universalism, at least in principle.

One aspect of the acceptance or rejection of a knowledge claim that Merton did not discuss was the scientist’s record of previous achievement, or the lack of it. When a scientist decides whether to further investigate a novel claim it is the plausibility of that claim, which depends, in part, on the credibility of the author of that claim which is an important, but certainly not the only, consideration.² One might also ask whether the reputation of the latter will influence whether their work is published. These are not independent issues. If a claim remains unpublished³ or is not further investigated, it has virtually no chance of being accepted as scientific knowledge.

How does the physics community deal with the subsequent work of a scientist whose earlier work has been regarded as incorrect? An interesting case of this involves Joseph Weber, who pioneered the development of an experimental apparatus designed to detect gravity waves, and who later claimed to have observed those waves. Weber’s claim was soon rejected by virtually all of the physics community, although Weber himself continued to defend his work. In the course of this defense Weber made a very unorthodox and startling suggestion regarding the scattering of neutrinos. Weber had connected his theory of the scattering of gravity waves, designed to show the increased sensitivity of his gravity wave antenna, to the scattering of neutrinos. In this essay I will briefly summarize the early history of gravity waves until the rejection of Weber’s claim around 1975, and follow Weber’s work on gravity waves until 1984 when he published his neutrino hypothesis. I will also examine the reaction of the physics community to that suggestion.

The Early History of Gravity Waves⁴

In the late 1960s and early 1970s Joseph Weber claimed that he had detected gravity waves (Weber 1969; 1970; Weber, Lee et al. 1973). One problem was that the rate of detection was far in excess, by a factor of 1,000, of what was expected by calculations based on Einstein’s General Theory of Relativity. Nevertheless, Weber’s results were sufficiently credible that several experimental groups attempted to replicate his findings. None were successful. In this case, the physics community was forced to compare Weber’s claims that he had observed gravity waves with the reports from six other experiments which failed to detect them. The results presented by Weber’s critics were not only more numerous, but they had also been carefully cross-checked. The groups had exchanged both data and analysis programs and confirmed their results. The critics had also investigated whether or not their analysis procedure, the use of a linear algorithm, could account for their failure to observe Weber’s reported results. They had also used Weber’s preferred analysis procedure, a nonlinear algorithm, to analyze their own data, and still found no sign of an effect. They had also calibrated their experimental apparatuses by inserting acoustic pulses of known energy and finding that they could detect a signal. Weber, on the other hand, as well as his critics using his analysis procedure, could not detect such calibration pulses.

There were, in addition, several other serious questions raised about Weber’s analysis procedures. These included an admitted programming error that generated spurious coincidences between Weber’s two detectors, possible selection bias by Weber, Weber’s report of coincidences between two detectors when the data had been taken four hours apart, and whether Weber’s experimental apparatus could produce the narrow coincidences claimed. It seems clear that the critics’ results were far more credible than Weber’s. Weber did not agree and offered various answers to the criticisms, which the physics community did not and plausible or compelling. By 1975 a consensus had been reached that Weber’ claim was unsubstantiated.

The fact that the physics community had reached such a consensus did not mean that the investigation of gravitational radiation ceased. Gravity waves are an important prediction of Einstein’s General Theory of Relativity⁵ and the experimental search for them has continued to this day (See Collins 2004). It also did not mean that Weber stopped working on the subject. Collins has discussed both Weber’s subsequent funding history and given an account of some of the later work on gravity waves by both Weber and others who joined in his defense. Weber’s later work was not totally ignored, although it certainly did not attract the attention of his earlier claims.⁶

More on Gravity Waves

In 1976 Weber and his collaborators (Lee, Gretz et al. 1976) published a detailed answer to some of the criticisms offered of his earlier work.⁷ Weber had previously offered a rather unusual explanation of why gravitational wave detectors might be more sensitive than others believed and thus might explain the high flux of gravity waves that he had detected. He noted that

Expressions for the antenna cross-section [sensitivity] require very reasonable assumptions and approximations that have not been tested by experiment. It is also possible that the antenna is operating in a more sensitive mode than ordinarily assumed. Frozen-in metastable configurations within each detector might decay to equilibrium as a result of collective excitation by gravitational radiation, releasing far more energy than implied by the gravitational-radiation flux. (Weber 1970, pp. 183–84)

Weber did not comment on why this would not have resulted in the detection of a higher gravity wave flux by his critics. He presumably thought that this was due to their use of a linear analysis algorithm, which he believed was inferior to the nonlinear algorithm he used (See discussion below).⁸

This speculation was, in fact, explicitly tested by Ronald Drever and his collaborators (Drever, Hough et al. 1973). They tested it in two different ways. They first injected below threshold pulses into their detector to see if Weber’s proposed mechanism would make them become observable. It didn’t. “In a run lasting two months, none of the small pulses became detectable” (p. 341). The second test involved injecting a train of five equal-amplitude, above-threshold pulses. “One might have expected any amplification process to be far more effective for the first pulse of a train than for the last, if the recovery of stored energy in the bar is a slow process and the first pulses in a train have depleted the accessible energy. We in fact see no significant change in the observed pulse height” (p. 341). They concluded “that there is no important multiplication process in this detector” (p. 341).

One of the most important aspects of Weber’s 1976 defense was his discussion of signal thresholds. Weber had been accused by his critics of manipulating his threshold for a gravity wave signal in order to maximize his result.⁹ Weber had denied the accusation, and in this paper he presented evidence that his results were, in fact, independent of the threshold used. Weber began with a discussion of the importance of setting an appropriate threshold.

An important aspect of the search window is the set of thresholds employed for a coincidence experiment.¹⁰ Suppose there are small numbers of moderately strong signals. Setting thresholds too low will give a very large accidentals rate with large fluctuations which mask the signal. Setting thresholds too high will result in no coincidences at all. (Lee et al., 1976, p. 895)

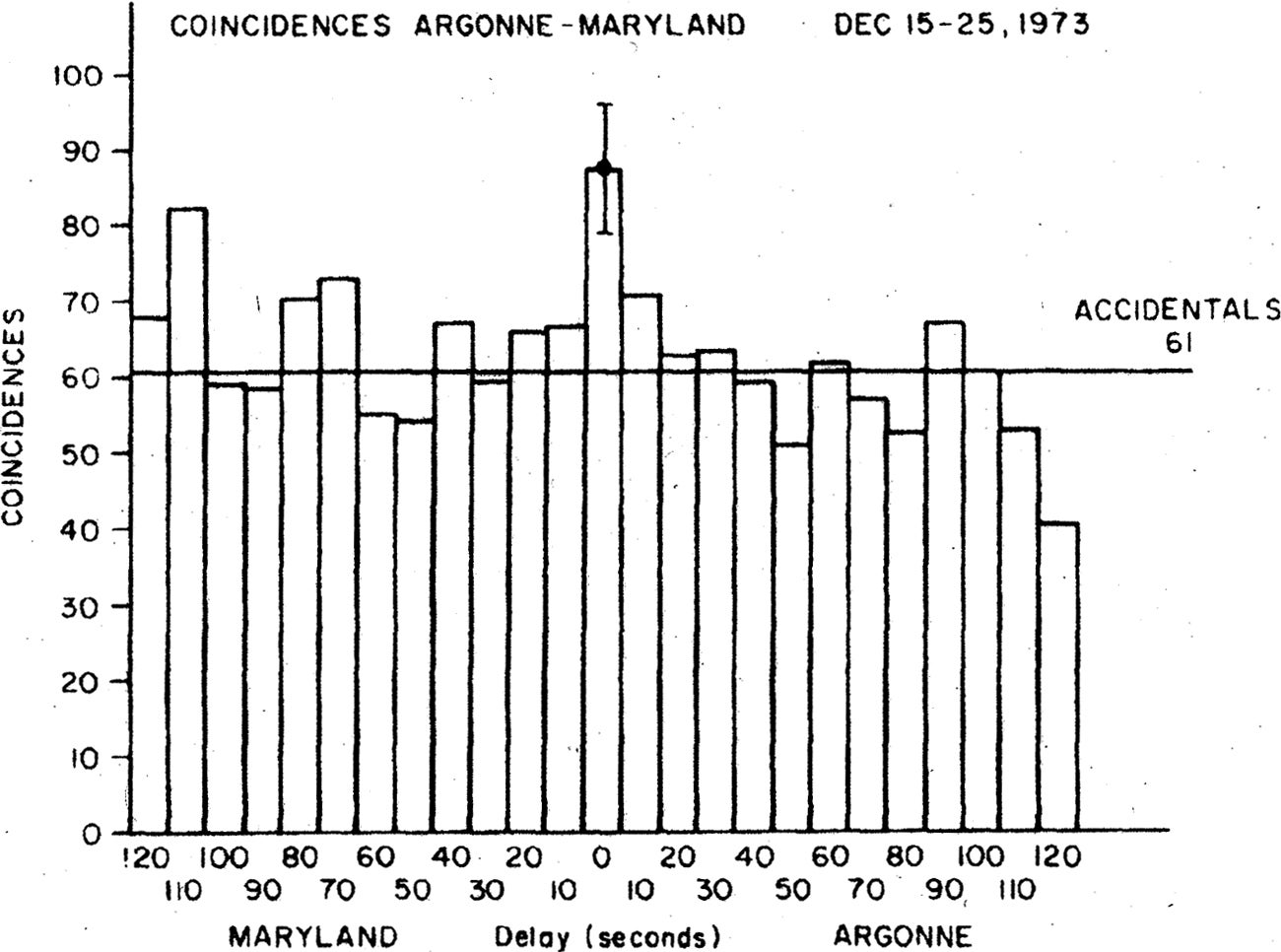
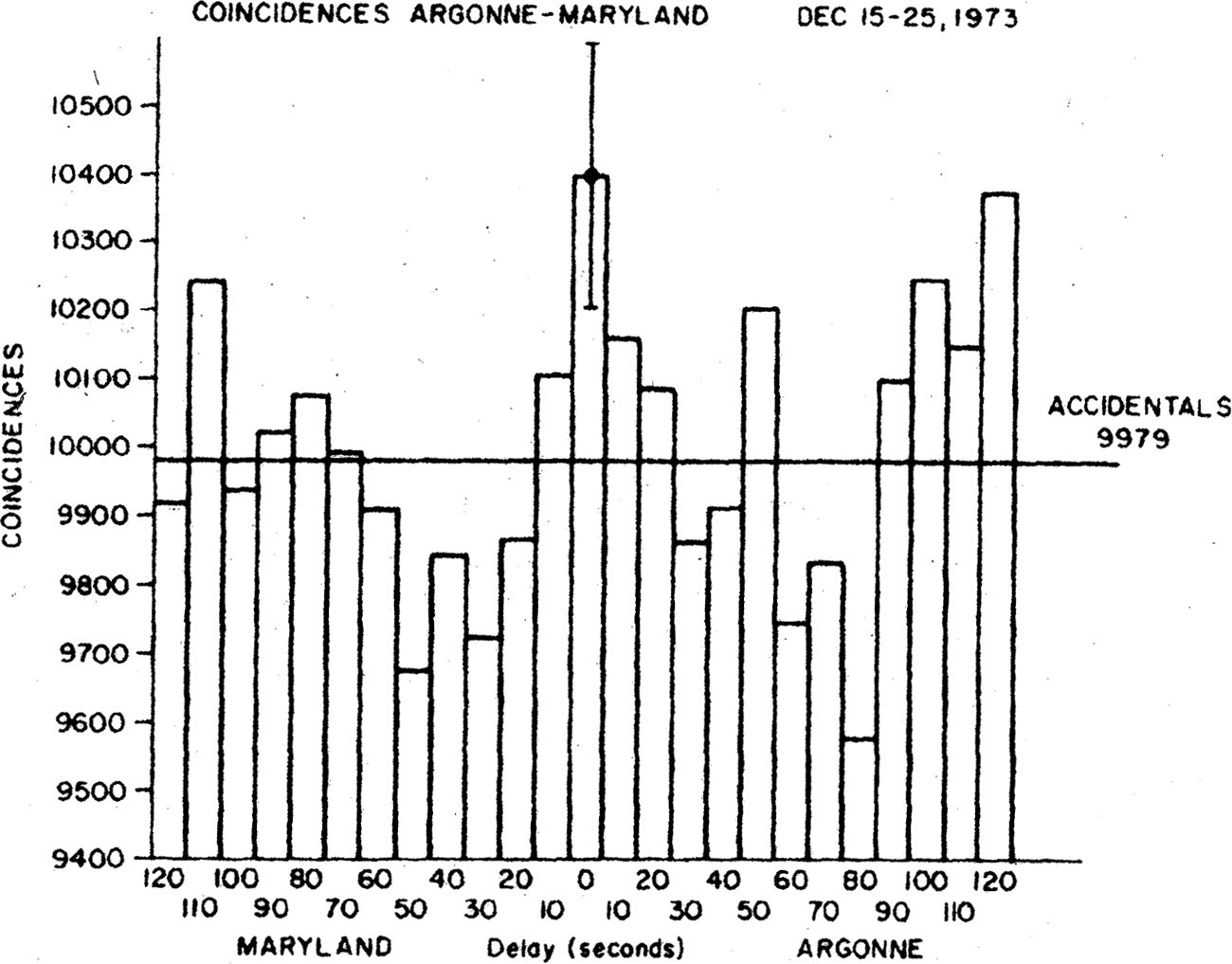
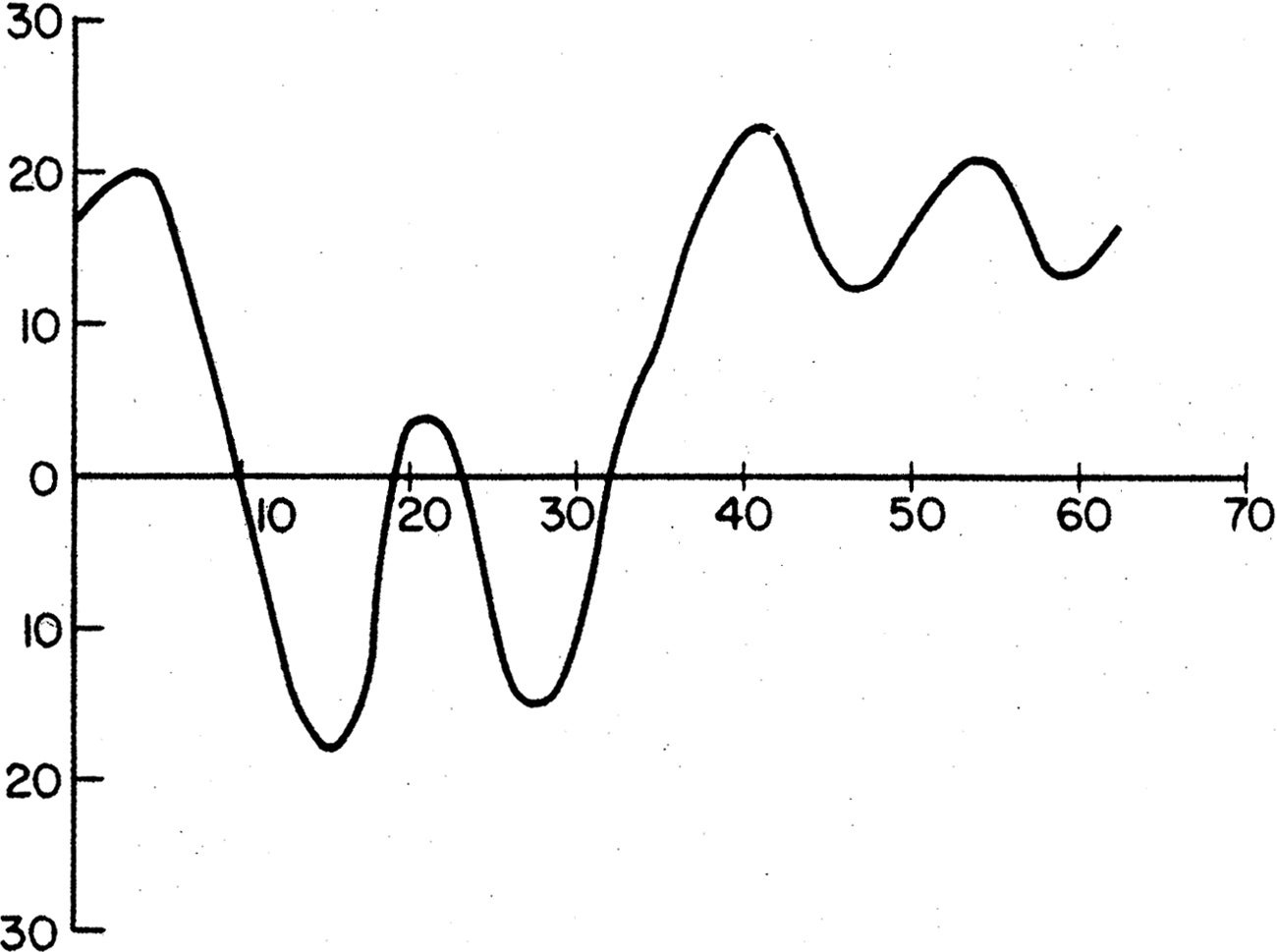
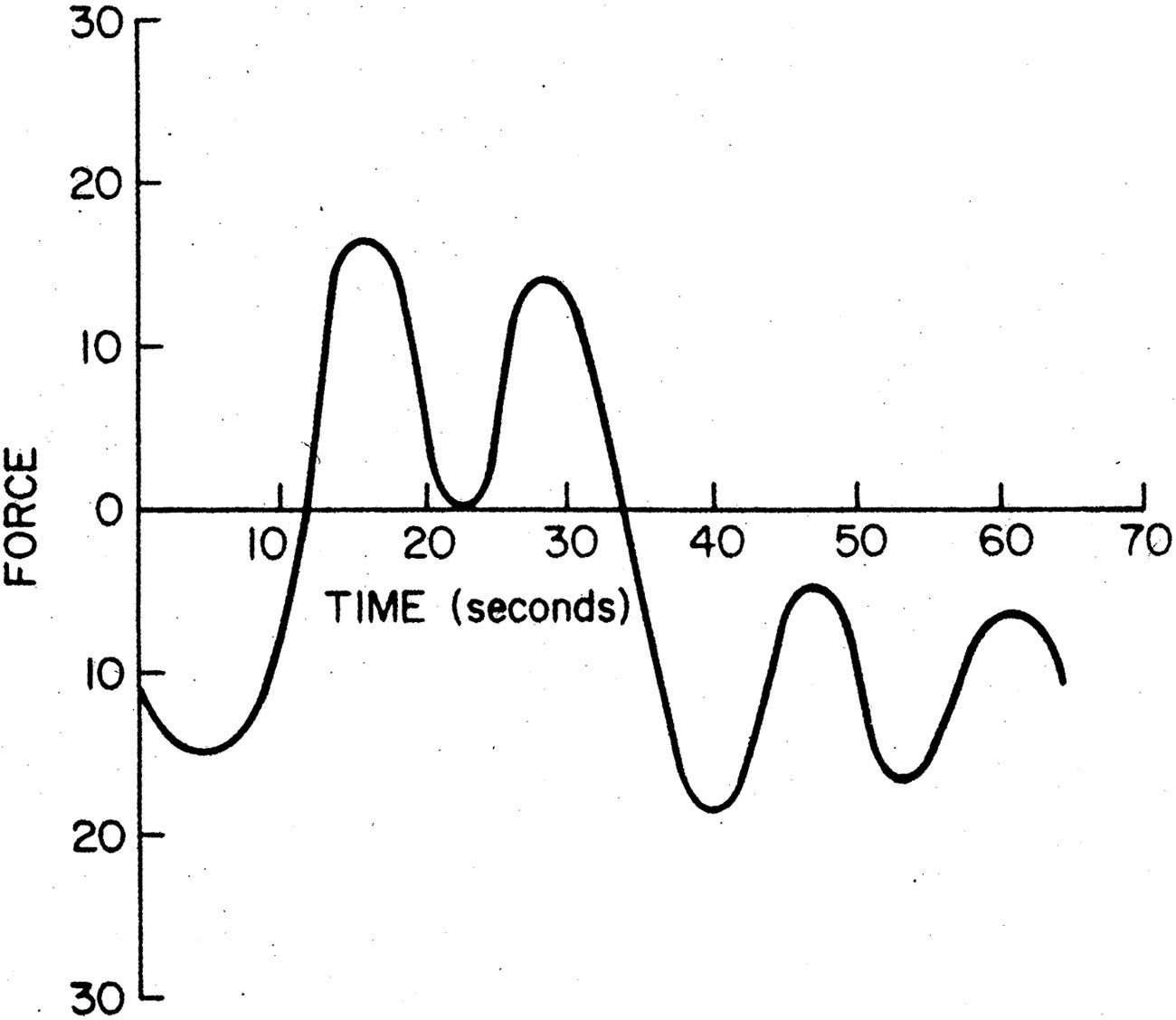
Weber then presented his data as a function of threshold. Figures 1 and 2 show his result for the lowest and highest threshold, respectively. He concluded that the signal was consistently present.

Figures 2–8 show the effects of changing threshold, with the largest accidentals rate ~10,000 [the highest rate] giving a zero-delay excess of 2.1 standard deviations. Raising the threshold and lowering the accidentals rate to 1,069 gives a zero-delay excess of 3.0 standard deviations. … An accidentals rate 62 of gives a zero-delay excess of 3.0 standard deviations. … Thus, a variation in accidentals rate by a factor of 16 gives a zero-delay excess exceeding 
3 standard deviations, and a range of 4 to 1 in accidentals rate gives a zero-delay excess greater than 4 standard deviations. (Lee et al., 1976, pp. 895–896)

A second important point concerned the choice of analysis algorithm. Weber noted that for his preferred nonlinear algorithm “A single excitation of the antenna which produces a large V_NB [signal] may cause during the long relaxation time a number of output pulses which will exceed threshold …” (p. 894; Figure 3). This phenomenon of proliferation, he argued, would give rise to a larger signal for the nonlinear algorithm as compared to the linear analysis algorithm favored by his critics. “For ex- ample, suppose there are a few large pulses causing proliferations … for the nonlinear algorithm A. A single pulse may result in a large number of threshold crossings giving several coincidences for algorithm A but only one for algorithm B [linear]” (p. 899). In fact, Weber admitted that there were “extended periods when the algorithm A yields a zero-delay excess larger than 4 standard deviations, but algorithm B yields essentially nothing” (p. 897). Collins cites a letter from James Levine, one of Weber’s critics, in which Levine pointed out that the proliferation effect, should have exactly the same effect on both noise and signal, without affecting their relative size in any way (Collins 2004, p. 198).

Weber had previously admitted that the linear algorithm was better at detecting the short pulses that most physicists believed would be characteristic of gravity waves. The proliferation effect led him to conclude that the actual gravity wave pulses were longer than his critics expected.¹¹

Weber continued his defense against possible human bias in a 1977 letter to Nature in which he outlined the steps he had taken to do so (Weber 1977). These included removing himself from direct participation in data reduction in 1972, checking that both the computer and human analyses of the data gave the same results, and showing that spurious signal injected into the data stream from time to time would be detected.

Collins reported that, except for self-citations to Weber’s own work, these papers (Lee et al. 1976 and Weber 1977) were subsequently cited only once each. This is not quite correct. During the period between 1977 and 1981, the date of Weber’s next refereed publication, these papers were cited seven times.¹² To be fair, none of these comments was very positive.

Most importantly, no attempts were made to answer Weber’s most recent defense of his results or to discuss his claim that his new experimental results had been shown to be independent of the pulse threshold used.¹³ Weber’s results on gravity waves were simply regarded as incorrect. The fact that no one within the physics community thought it worthwhile answering Weber’s defense indicates that he had lost all credibility as far as gravity wave experiments were concerned. As Collins remarks, “In terms of their impact they [Weber’s 1976 and 1977 papers] might just as well not have been written” (Collins 2004, p. 201). As we shall see, however, his later work on neutrino detection was taken seriously enough to be worthy of both comment and criticism and of additional experimental work by others.

Weber’s papers were cited in other reports of attempts to detect gravitational radiation. Most of the papers concluded that Weber’s results were incorrect. A typical comment came in the 1978 review of gravitational wave astronomy by J. Anthony Tyson and R. P. Giffard. “The giant bursts observed by Weber have not been detected in careful searches by several other groups of observers” (1978, p. 521). “It must be concluded that the interpretation of the Weber events as gravitational wave pulses is erroneous, since there is no corroborated evidence either for an excess number of coincident events or any sidereal correlation” (pp. 544–545).¹⁴ A similar view was expressed in Kafka and Schnupp (1978). “The aim had been to verify or exclude the existence of gravitational wave pulses of the kind proposed by Weber as an explanation for his own results. Although the non-existence became obvious a long time ago, it seems appropriate to publish our final negative result, because our experiment was as similar to Weber’s as possible, whereas all other experiments deviated in one way or the other. Moreover, we think we have set the lowest limits obtained by Weber-type experiments over a reasonably long period of observation” (p. 97, emphasis added). Kafka and Schnupp did, however, address the question of the choice of analysis algorithm even though they believed that the issue had already been settled. They applied two analysis algorithms to their data, S_A, the linear algorithm, and S_E, which “was a reasonable approximation to Weber’s preferred algorithm” (p. 101). “The results do not give the slightest hint of a simultaneous influence in both detectors. If the significant observations reported by Weber’s group … had been due to gravitational radiation of any kind, they should have shown even more significantly in our experiment” (p. 102). O.G. Jensen, who was not an experimenter in the field, but who was considering the possibility of seismic detection of gravitational radiation was more neutral. “It is now universally accepted that the possibility of gravitation radiation is inherent in Einstein’s theory of gravitation, General Relativity. In recent years, much effort, both experimental and theoretical, has been devoted to the detection of such radiation. However, success has not been notable. Weber, perhaps the foremost proponent of such research, has offered evidence suggesting that certain coincident excitations of his two, well separated, mechanical antennae, could have been caused by gravitational radiation bursts in the kilohertz band. However, many other researchers in this field … have been unable to verify Weber’s observations” (Jensen 1979, p. 2057).

Weber’s results were, however, cited to justify further research into the sensitivity of gravitational wave detectors and for the building of more sensitive detectors. Thus Giffard, after discussing the disagreement between Weber and his critics, stated that “In view of such results, it is desirable to consider in detail how the sensitivity of the antennas can be improved, …” (Giffard 1976, p. 2478). Tyson and Giffard remarked that, “The need for more sensitive detectors is clearly established by the lack of confirmation of Weber’s exciting results” (Tyson and Giffard 1978, p. 545).¹⁵

I also note the very positive statement concerning Weber’s initial contributions to the field of gravity waves by Tyson and Giffard. “It is clear that none of this research would be occurring were it not for the pioneering experiments of Joseph Weber, who gave birth to the field a decade ago with the first operating gravitational wave detector. His aluminum bar antenna is the prototype of nearly all recent detectors” (1978, p. 522).¹⁶

In 1981 Weber suggested another possibility for enhancing the sensitivity of a gravity-wave antenna. This was the possibility of preparing the antenna in a correlated initial state. “This suggests the possibility of preparing an antenna in a quantum state very different from thermal equilibrium state [the usual state of such an antenna]. Such a state might be maintained for a significant fraction of observation times. Here it is shown that an appropriate choice of quantum states may give an enormous increase in the energy absorbed from gravitational radiation” (Weber 1981, p. 542). Weber admitted, however, that “it is by no means obvious that an antenna can be prepared in such states, and that the unwanted interactions can be kept sufficiently small to result in major improvements in signal to noise ratio. The possibilities of such improvements are now being studied” (p. 544).¹⁷ Weber’s theoretical conclusions were supported by Walls and Zoller (1981). They noted that Walls had previously suggested a similar scheme to increase the sensitivity of a cyclotron resonance detector to detect infrared radiation and concluded that “… we have shown that the use of correlated quantum states leads to a possible large increase of the sensitivity of gravitational antennas” (p. 120). Weber’s suggestion was also considered by Dododnov, Manko, and Rudenko (1982; 1983). They, on the other hand, concluded that Weber’ scheme would not increase the sensitivity of the gravity wave antenna, although they suggested that another quantum mechanism could have such an effect.

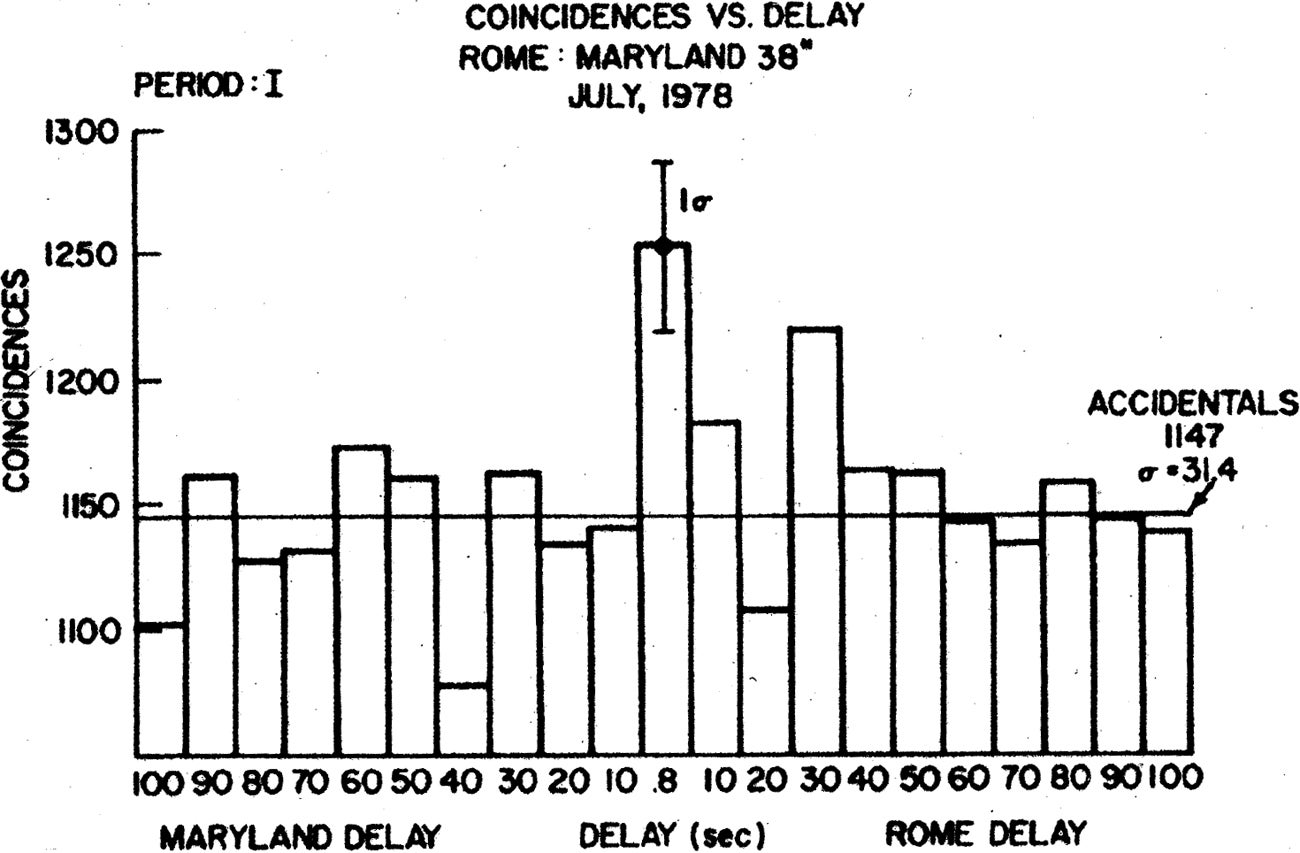
Weber (1984) continued his defense of his gravity wave results, this time invoking the theory of coherent scattering. He remarked that, “For an extended period, other observers were not able to find the background of pulses. However, beginning in 1980, all of the Maryland observations were confirmed” (p. 1187).¹⁸ In support of this statement he cited the results of a Maryland-Rome collaboration (Ferrari, Pizzella et al. 1982), of which Weber was a member, and a result from a group at Stanford (Boughn, Fairbank et al. 1982). The Maryland-Rome collaboration concluded that there was a positive signal at zero time delay between their two antennas. “The histograms are evidence for an external background of signals [a real gravity wave signal], greatest during the first period, and having a level of confidence associated with 3.62 standard deviations” (Ferrari, Pizzella et al. 1982, p. 2486). The qualification that the signal was greatest for the first period was important. The group had taken data during the period July 5–13, 1978. They divided that interval into three periods: Period I, July 5–8; Period II, July 8–11; and Period III, July 11– 13. As the group noted, a significant signal (3.56 standard deviations) was seen only during Period I (Figure 4). No significant signal was observed in the other two time periods, although a signal was also seen when the data for all three periods were combined (Figure 5). They also noted that effects of a similar magnitude were seen at non-coincidental times. They remarked, “To summarize, the coincidental effect is observed to be the largest in one of the three periods when these periods are viewed separately. In addition, effects of a similar magnitude are seen at non-coincidental times. When the periods are combined into two contiguous groups, the coincidental effect is the largest in the first group at 3.62 σ with no other delay in either group producing an excess above 3.0 σ. And finally, when all the data are combined, the largest excess is again coincidental” (p. 2479).

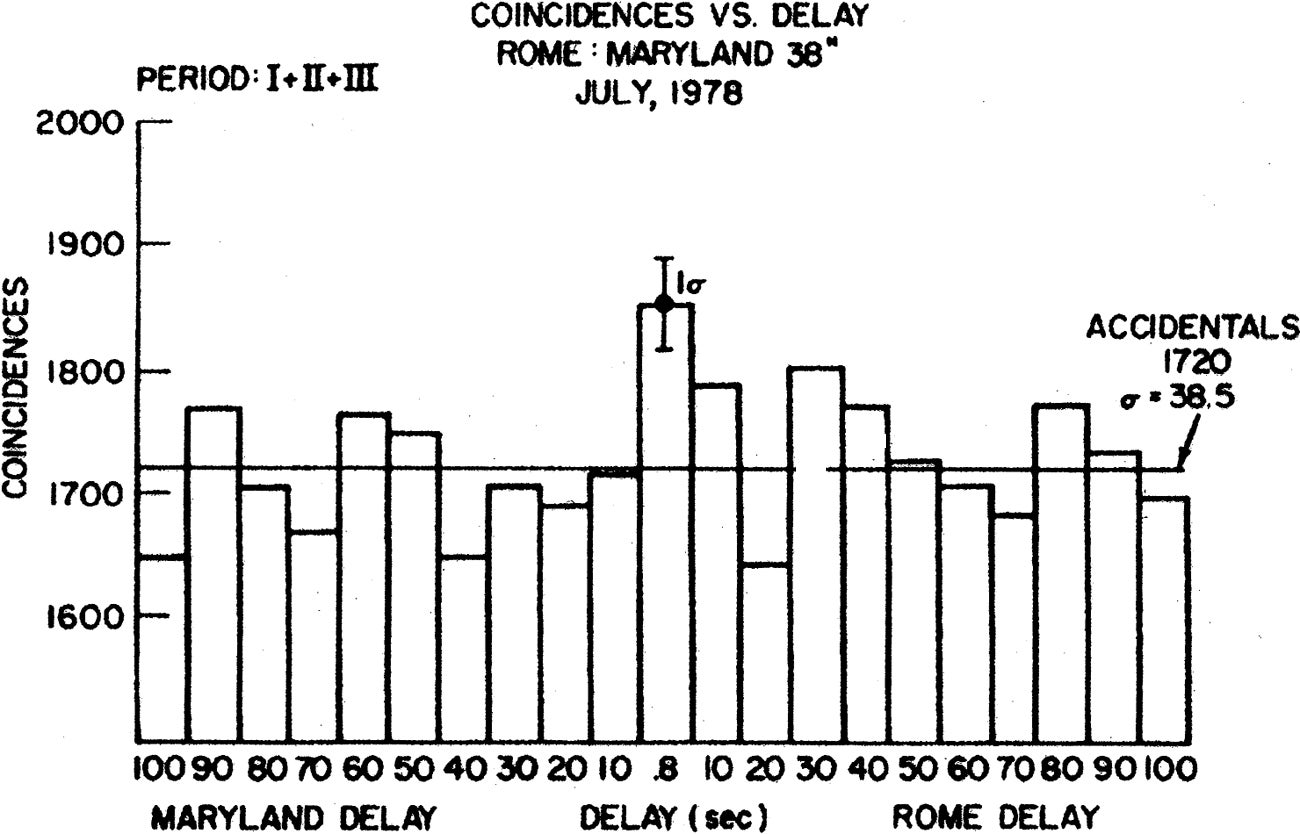
Weber also noted that the Stanford group had reported 8 events with magnitude greater than 10 K. (The noise signal expected would have an average value of 1.5 K.) That was correct, but it is important to note that the Stanford group made no claim that they had seen gravitational radiation. Their conclusion was that, “The data from about 74 days of operation have been used to place a new observational upper limit on the distribution of wide-band gravitational wave pulses which reach the earth during a typical period” (Boughn, Fairbank et al. 1982, p. L22). Weber also cited a letter from Pizzella who wrote, “a preliminary analysis on your data showed also a sidereal effect” (Weber 1984, p. 1187). That effect subsequently disappeared and had, in addition, initially shown a 24-hour period, when a 12-hour period was expected.

Weber’s results were contradicted by Brown et al. (1982) who, in a 440-day experimental run detected only “one clearly nonthermal event. This event was apparently of local origin, based on the analysis of coincident data from the far more sensitive Stanford cooled antenna. … This search exceeds previous published searches in sensitivity, and sets a 90%-confidence upper limit over the 440-day duration of the experiment of < 5 x 10^-3 events per day above 114 GPU … (1 gravitational pulse unit 10⁵ erg cm^-2 Hz^-1). These results confirm the previously reported null results to a much lower event rate” (p. 1216).

As discussed by Collins (2004), Weber continued to receive funding for his gravity research and continued publishing on gravity waves, although most of his subsequent work appeared in non-refereed conference proceedings rather than in refereed journals.¹⁹ It was also certainly not the end of searches for gravity waves, which have continued to the present with low temperature antennas and subsequently with even more sensitive interferometers (See Collins 2004 for details).

Neutrinos and Antineutrinos

Weber’s 1984 paper also contained a startling new theoretical suggestion concerning neutrino detection, as well as experimental results which supported that hypothesis. Weber, assuming an infinitely stiff crystal, calculated that the coherent scattering of neutrinos from such a crystal would vary with N², where N was the number of scatterers.²⁰ For a macroscopic crystal N would be a very large number²¹ and this would increase the very small neutrino scattering cross-section by many orders of magnitude. This would mean that instead of using a tank containing 100,000 gallons of cleaning fluid,²² one could use a sapphire crystal that one could hold in one’s hand as a neutrino detector.

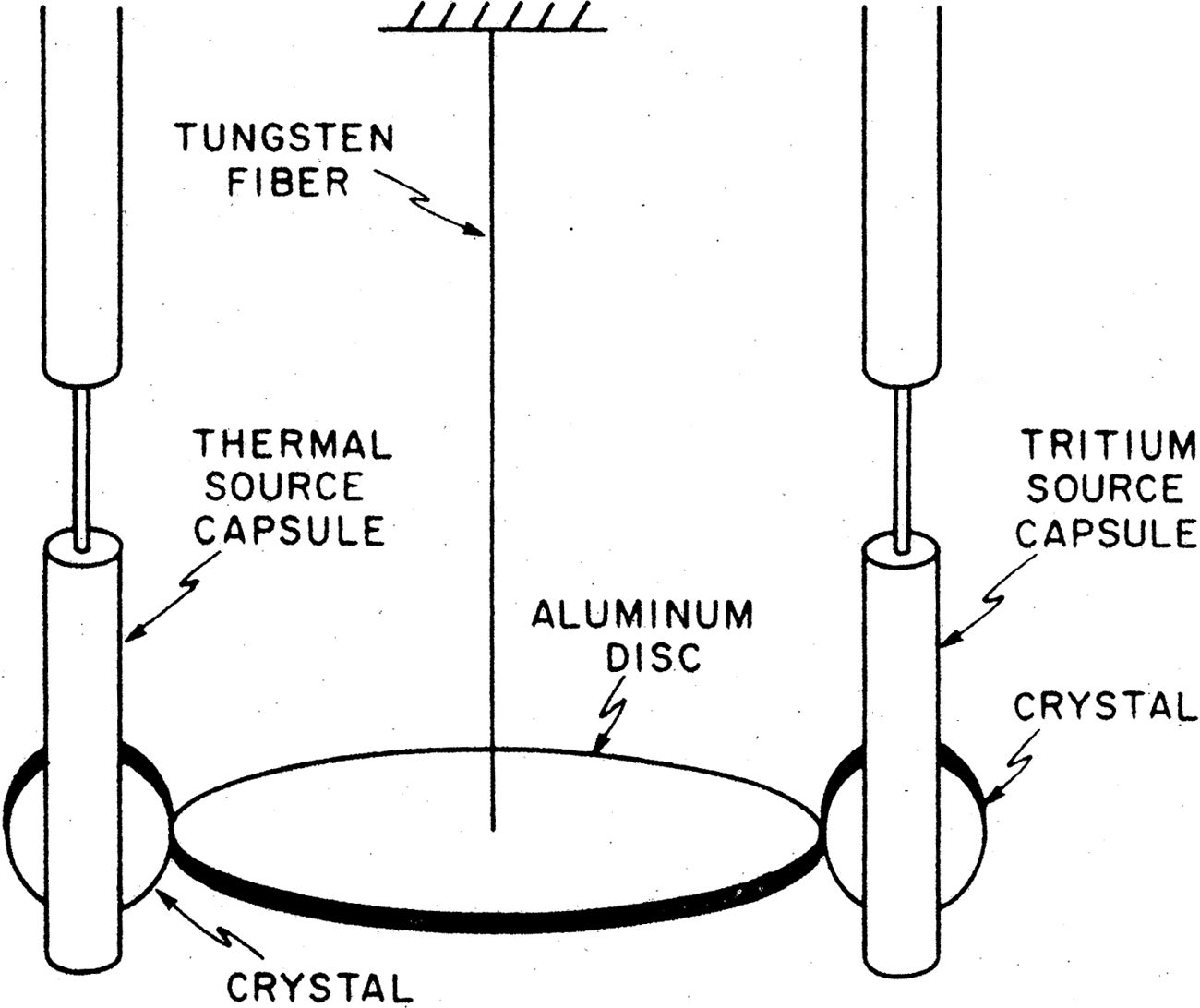
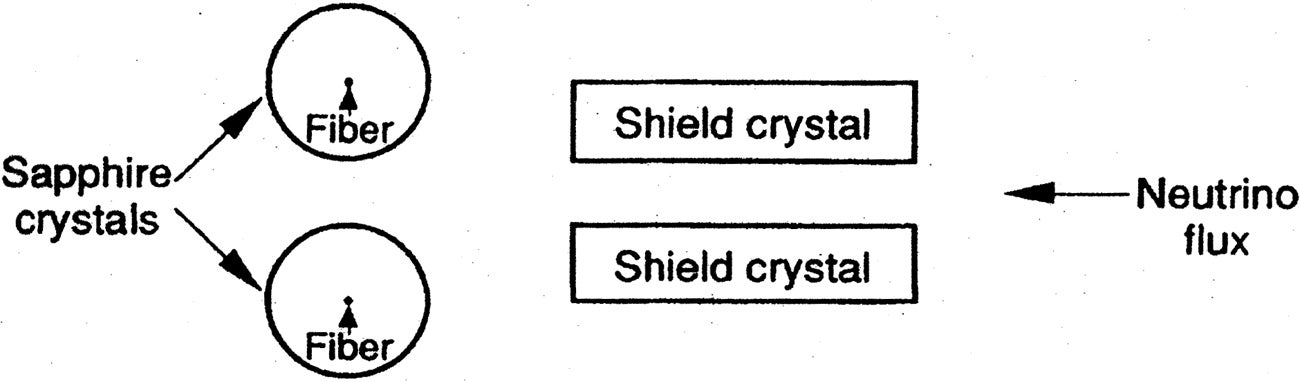
Weber also presented experimental results in support of his coherent- scattering hypothesis. The apparatus is shown in Figure 6. The torsion balance contained two sapphire crystals, 2.5 cm in diameter and 0.38 cm thick, attached to an aluminum disk. There were two identical aluminum cylinders. A 3000 Curie titanium tritide radioactive source was lowered into one cylinder and an equal mass dummy source was lowered into the other cylinder. If there were a significant force due to the neutrinos emitted by the tritium, the balance would rotate away from the tritium source. “The torsion balance shown in Fig. [6] was employed for measurement of the momentum transferred to the crystal by antineutrinos. A closed loop servosystem was developed to measure the forces exerted on the crystal. A radio frequency bridge became unbalanced whenever the torsion balance was displaced from its equilibrium position. The unbalance voltage was then amplified and then employed to produce an electrostatic force to restore the balance to its equilibrium position. The force is measured by observing the output voltage” (Weber 1984, pp. 1197–98). Weber was quite careful about possible backgrounds that might simulate the effect he was investigating and remarked that

A 3000 Ci [Curie] tritium source generates 0.1 W as a result of the kinetic energy of the 6-keV decay electrons. A torsion balance will respond to a heat source for several reasons. Thermal gradients may result in unbalanced gas pressures; thermoelectric effects may generate potentials. In order to make the thermal effects as small as possible, the cylinders in which the tritium source moved were enclosed in several layers of superinsulation. It was observed that the thermal effect has a relaxation time of several minutes. To reduce the thermal contribution, the servosystem [used to detect any deflection of the balance] was designed with a response time of 9 sec. It was also observed that the sign of the thermal response was opposite in the two cylinders for raising and lowering the tritium source and the dummy. An electrically heated resistance was installed in the dummy. Observations then indicated that the thermal response force was less than 10% of the antineutrino forces in these experiments. Since the masses of the tritium source and dummy capsules were equal, the gravitational force of the tritium source was also balanced out. (Weber 1984, p. 1198)

The torsion balance was calibrated by replacing both the dummy and the tritium source with a lead mass in one side of the balance. “The gravitational interaction of the lead mass and the target crystal provides a known force to calibrate the torsion balance in terms of the servosystem output voltage” (p. 1198).

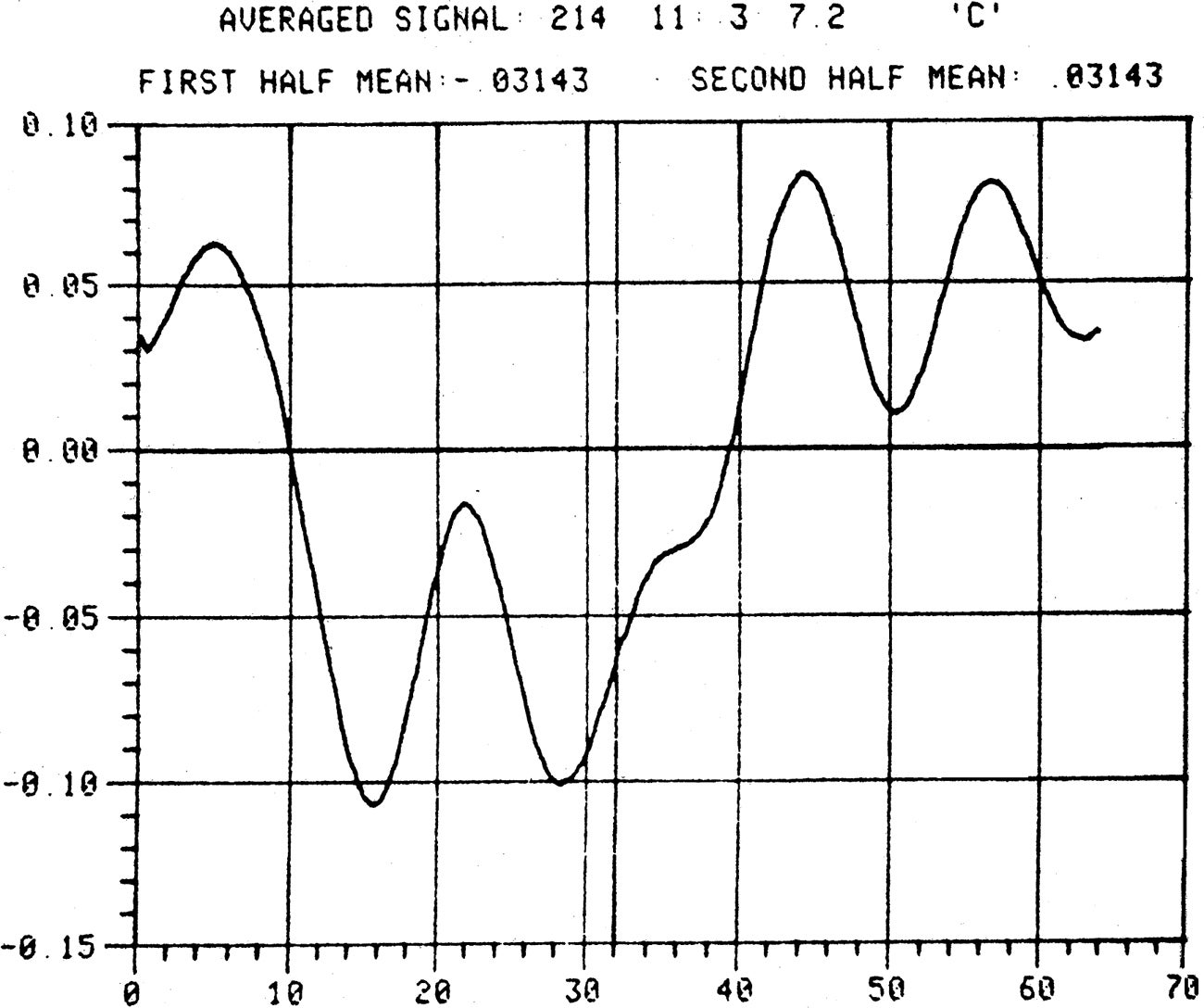
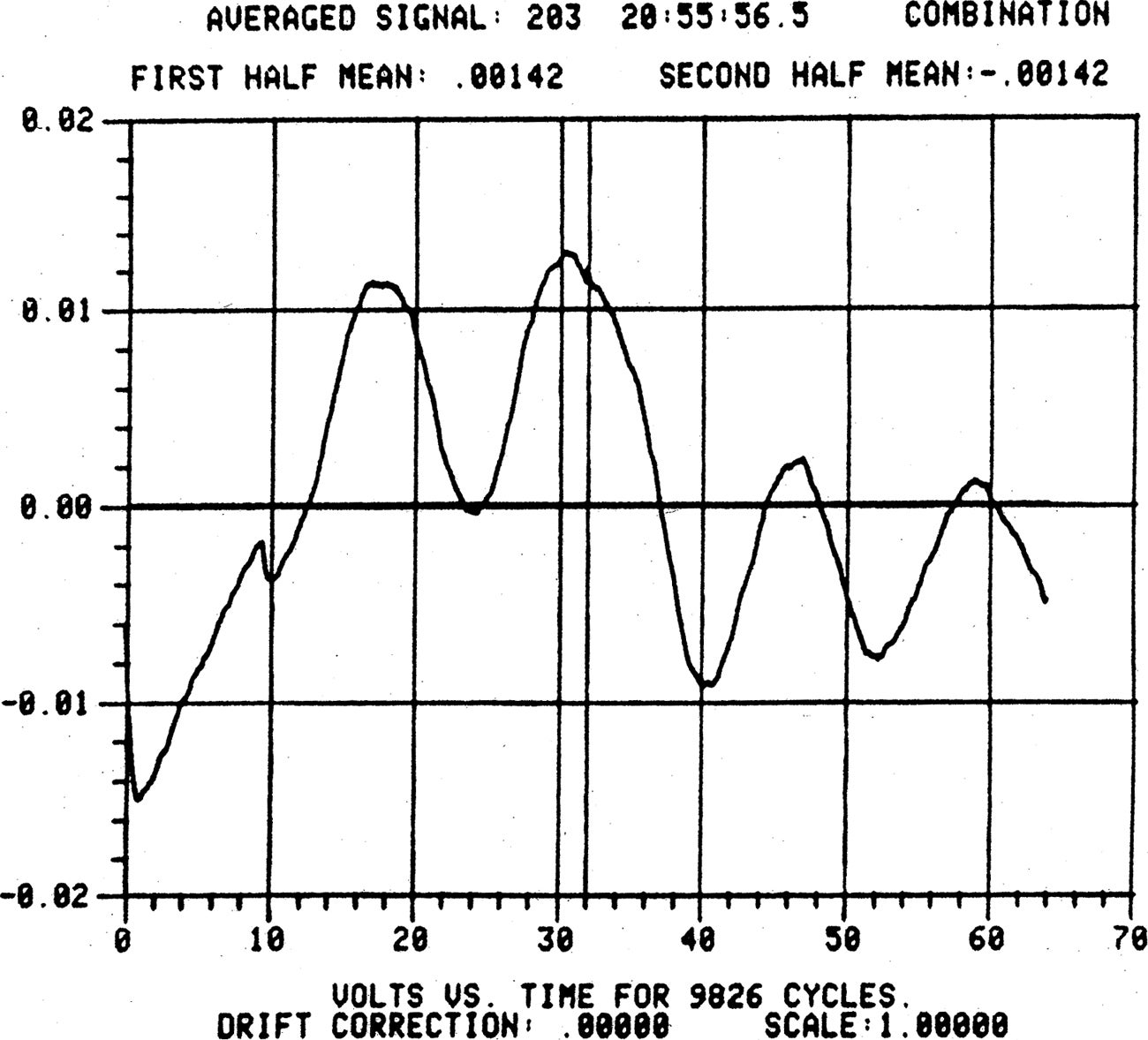
The output voltage for the gravitational interaction of the lead mass with the target crystal, an attractive force, is shown in Figure 7. Figure 8 shows the output voltage for the interaction of the tritium source with the target crystal, expected to be repulsive. They clearly show opposite effects, as expected. The predicted results are shown in Figures 9 and 10, and are in agreement with the observations. Weber calculated a scattering cross-section of 1.06 ± 0.44 cm² and remarked that “It is noted that this is a ‘blind’ experiment. All data are on a magnetic tape which is processed by a programmer who has no information concerning what is expected. Figures [7] and [8], without corrections, imply that a repulsive force is being observed consistent with a large scattering cross section” (p. 1203). Weber’s comments were intended to avoid any repetition of the accusation that he had manipulated his analysis procedure to maximize his expected effect.

Weber concluded that “Cross sections are now available for coherent low-energy neutrino and antineutrino experiments exceeding the incoherent values by more than 20 orders. Gravitational radiation antenna cross sections for some quantum processes exceed the predicted classical values by more than eight orders. These phenomena provide new frontiers for gravitational and neutrino astronomy” (pp. 1206–7, emphasis added). If Weber’s results were correct, this would be an understatement.

Weber (1985) extended his calculations and presented additional experimental results in support of his suggestion. He reported observing both a heating effect due to inelastic coherent scattering of antineutrinos as well as an elastic cross section of 1.5 cm² for a 100 g crystal. Both experiments used antineutrinos from a nuclear reactor. A cross section for elastic scattering of antineutrinos from a 600 Curie tritium source on a 12 g crystal was also reported. No details of the experiments were provided but Weber promised that, “These experiments will be described in detail in forthcoming papers” (p. 1473).²³

A. Theoretical Objections

Weber’s calculations and experimental results on neutrino scattering were deemed plausible enough to be commented on by several authors. The comments were of two types. The first argued on theoretical grounds that Weber’s calculations were incorrect. The second pointed out that there were already experimental results that excluded Weber’s hypothesis. T.H. Ho (1986), for example, remarked that some neutron scattering experiments satisfied the conditions that Weber had required for his coherent effects. “Thus, in a silicon-neutron diffraction experiment, one should observe such a large coherent scattering cross section! Since such kinds of experiments have been done frequently and no one has found such coherent enhancement of neutron scattering, it seems that Weber’s mechanism could already be excluded by the present neutron diffraction experiment” (p. 295). G.F. Bertsch and Sam Austin (1986) argued that Weber’s calculation was incompatible with wave mechanics, and that one would expect to find an analogous effect in x-ray scattering. If Weber was correct then x-ray scattering on amorphous and crystalline silicon would be quite different whereas, “In fact there are negligible differences in the attenuation of x rays by amorphous or crystalline silicon, for thin alms of the same areal density” (p. 361).

M.N. Butler (1987) also discussed inconsistencies in Weber’s derivation and concluded that, “we have shown that Weber’s derivation of large cross sections is wrong on the basis of elementary physical arguments and that it is the result of an incorrect mathematical derivation” (pp. 1164–65).²⁴ He further noted that such coherence effects could only be observed if the wavelength of the neutrinos is comparable to the size of the crystal, an ultra-low-energy region.²⁵ Casella (1986) considered the scattering of neutrinos with wavelength to the lattice spacing of an ideal crystal, or considerably less.²⁶ He found that “The coherent force produced by neutrinos of such wave-lengths is linear in N. Hence, in agreement with Butler,²⁷ I and that a coherence factor O(N²) can occur only for neutrinos with ultra-long wavelengths” (p. 42).

Weber’s experimental result was considered “remarkable” by P.F. Smith (1987). He remarked, however, that “The purpose of this letter is to point out that the conventional treatment of coherent scattering in a rigid target … predicts a coherent neutrino force which is 23 orders of magnitude smaller than the force calculated (and apparently observed) by Weber” (p. 107). He concluded

Thus, since it appears physically impossible for particles of wavelength ~10^-8 cm to be scattered in phase (and with significant momentum transfer) from a volume 1 ~cm³ as conjectured by Weber, we must conclude that the observed repulsive force—agreeing in magnitude with Weber’s prediction—could not have been due to normal coherent neutrino interactions. It therefore represents either a totally new force or an experimental artifact of unknown origin which has coincidentally replicated Weber’s conjectured factor 10²³ enhancement. Clearly a more rigorous repetition of this preliminary measurement, with variation of some of the experimental parameters, would be required to distinguish between these two possibilities. (p. 109)

Weber’s suggestion was found “intriguing” by Y. Aharonov and his collaborators (Aharonov, Avignone et al. 1987). They noted that although Weber had presented experimental evidence in support of his hypothesis several arguments had been offered against the existence of such phenomena.²⁸ They also found that the force produced would vary linearly with N, the number of scatterers, not quadratically with N² as Weber had claimed. They did, however, remark that if the hypothesis were correct that several interesting consequences would follow. At this time the solar neutrino problem, in which the number of observed solar neutrinos was a factor of three smaller than that predicted by the Standard Solar Model, was still unsolved.²⁹ They remarked that

It might then be remotely possible that the distribution of crystal sizes conspires to reduce the count rate in Davis’s ³⁷Cl experiment by a factor of 3.

If this were the case, however, the total solar energy absorbed by the Earth would increase by ~20% over that due to photon absorption alone. One is tempted to wonder whether the present understanding of the Earth’s temperature due to solar photons and terrestrial radioactivity is complete enough to exclude such a revision; the change in the overall temperature due to solar neutrinos would be ≈15° C. (p. 1175)³⁰

Harry Lipkin (1987) also examined the question of coherent scattering from crystals and concluded that it “is to vary linearly with N for large N” (p. 1176).

B. Further Experiments

The promised details of Weber’s three experiments appeared in 1988 (Weber 1988). The first experiment reported used antineutrinos from a tritium source, which had an average energy of 6.2 keV. It was essentially the same experiment he had reported in 1984, with slight changes in both the apparatus and the analysis procedures, and with more data. He now reported a cross section of 2.05 ± 0.23 cm², in contrast to his previously reported preliminary result of 1.06 ± 0.44 cm². Weber noted, however, that his measured cross section differed from his calculated result by a factor of 10. Weber had an explanation. “Equation (2) gives a scattering cross section ~20 cm². This implies that most of the antineutrinos are scattered once by the first layers of the crystal. Multiple scattering will occur, and precise agreement with the single-scattering cross section is not expected. We believe that a much smaller crystal, with no dislocations, would have an observed cross section in agreement with (2)” (p. 37). In this experiment the radioactive tritium source was in close proximity to the detector and he thought it important to do other experiments that avoided problems of that experiment such as heating effects.

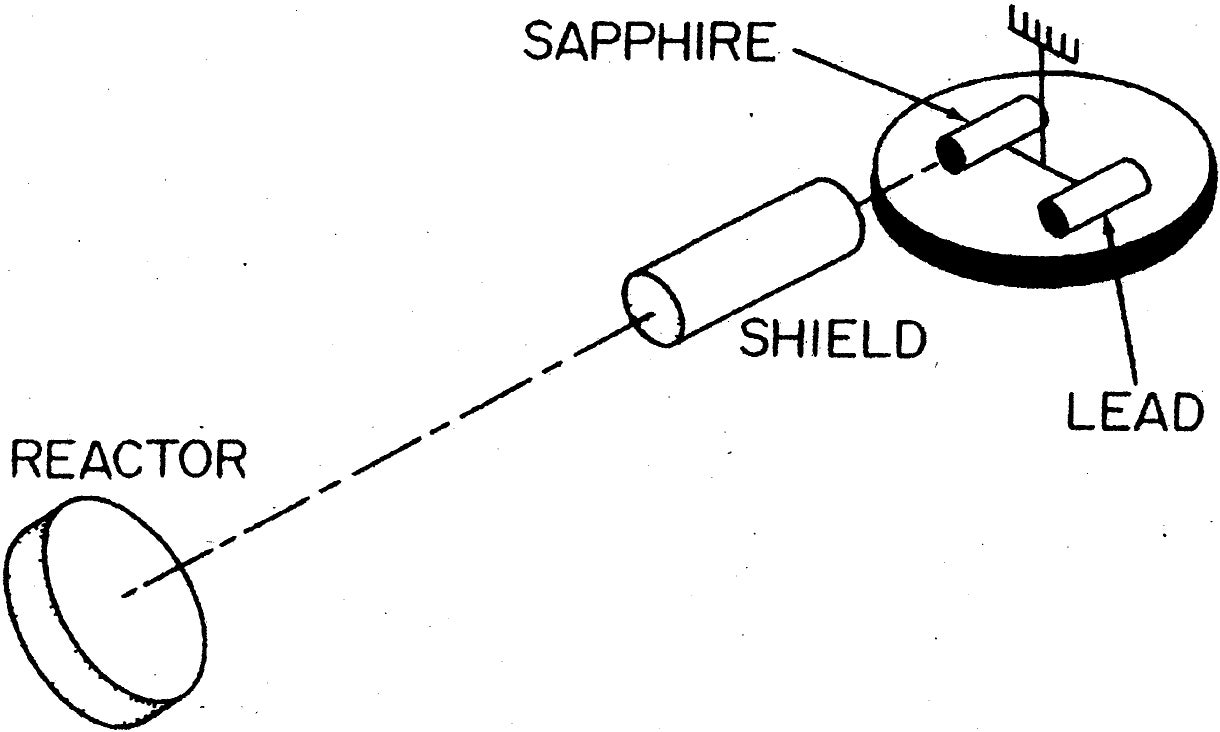
The second reported experiment used antineutrinos with an average energy of approximately 1.6 MeV from a nuclear reactor, in which the detector was quite distant from the reactor. The apparatus is shown in Figure 11. In this experiment Weber recorded the difference in the force on the torsion balance when the sapphire crystal detector was shielded by a 5 kg crystal and when it was not. Weber’s experiment depended on the correctness of his theory. “Again, if the large cross sections predicted by the theory are correct, a larger crystal should serve as an antineutrino shield. Switching is carried out by harmonically moving the shield into the line of sight between reactor and balance” (p. 37). He reported a force exerted on the balance of 3.9 ± 0.4 x 10^-5 dynes, comparison with his predicted value of 3.0 x 10^-5 dynes (Figure 12). “We are not stating that the theory as presented here is complete, and agreement of observation with theory is not claimed” (p. 37).

Weber’s third experiment used solar neutrinos, which avoided background and experimental problems associated with a nuclear reactor. In this case the torsion balance had a crystal on one side and an equal mass composed of lead sheets on the other (Figure 6).

If two equal masses having different composition are at opposite ends of a torsion balance, the Eötvös experiment is expected to give no observable torques, due to gravitational and inertial forces. However, if the masses have very different Debye temperatures, and very different crystal structures, the solar-neutrino flux is expected to produce torques.

A diurnal effect is predicted as the position of the Sun changes, relative to the balance. We have been observing the diurnal effect during the past two years, with a peak when the Sun is in the direction of the line normal to the line joining the two masses.

Considerable care is required to distinguish forces associated with solar neutrinos from effects of diurnal fluctuations due to variations in temperature, and laboratory building orientation. (p. 38)

The results for the solar-neutrino experiment are shown in Figure 13. Weber remarked that, “These data imply that a force of ~4.6 x10^-6 dynes is being observed, due to the elastic scattering of solar neutrinos” (p. 38).

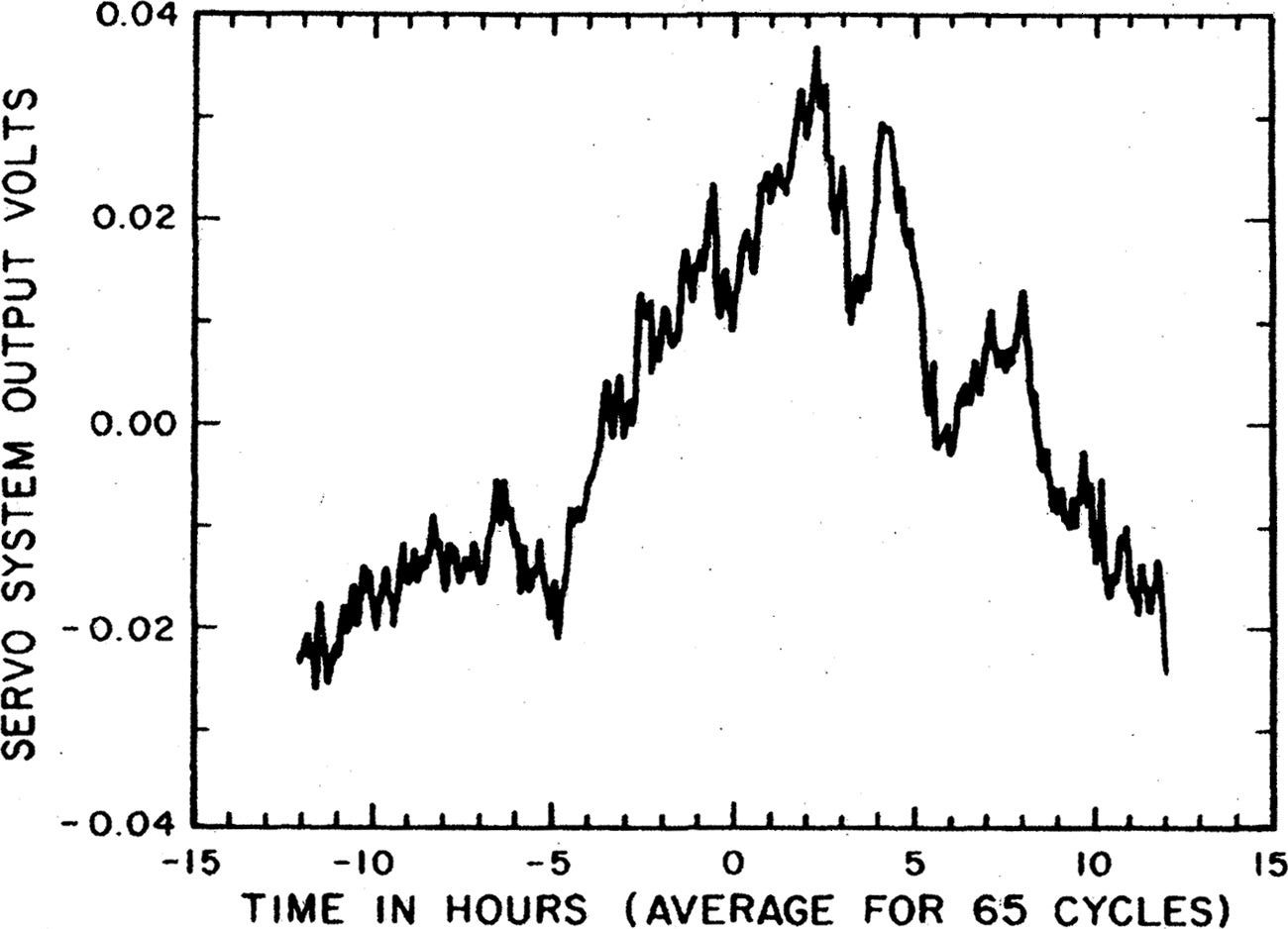
Weber concluded that, “Experiments with antineutrinos from tritium at about 12 keV, with reactor neutrinos at about 1.6 MeV, and solar neutrinos in the range 0 – 430 keV, give measurable forces, apparently due to coherent scattering from stiff nearly perfect crystals, consistent with the hypothesis of very large cross sections” (p. 39).

Weber’s theoretical calculations and, in particular, his experimental results on neutrinos were taken seriously by Franson and Jacobs (1992). The introduction to their paper noted that, “Although this [Weber’s] theory has been criticized by several authors, potentially important experiments should be repeated regardless of whether or not their results are understood theoretically” (p. 2235). They further remarked that Weber’s experimentally measured force from solar neutrinos corresponded to a transfer of 86 percent of the incident neutrino momentum to the crystal and that most of the neutrinos scattered at large angles. They incorporated this result into the design of their experimental apparatus (Figure 14). “The fact that nearly total neutrino scattering was apparently observed in the earlier experiments allows the possibility of using one crystal to shield the neutrino flux from impinging on a second crystal.³¹ … In view of this situation it was felt that it would be acceptable to use such a shield in the present experiment as a test of the earlier experimental results” (p. 2235). The authors remarked, however, that because of this assumption, care would have to be taken in the interpretation of their results.

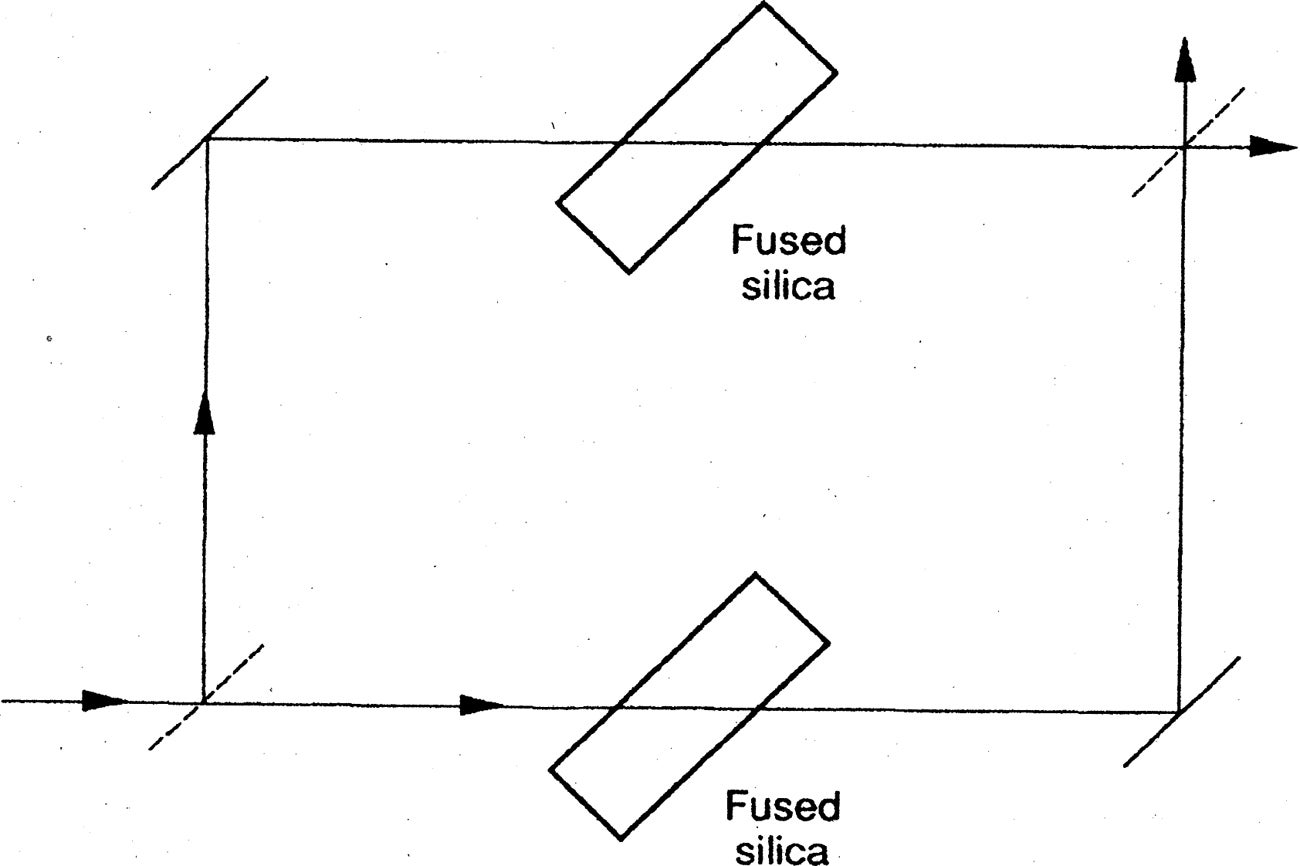
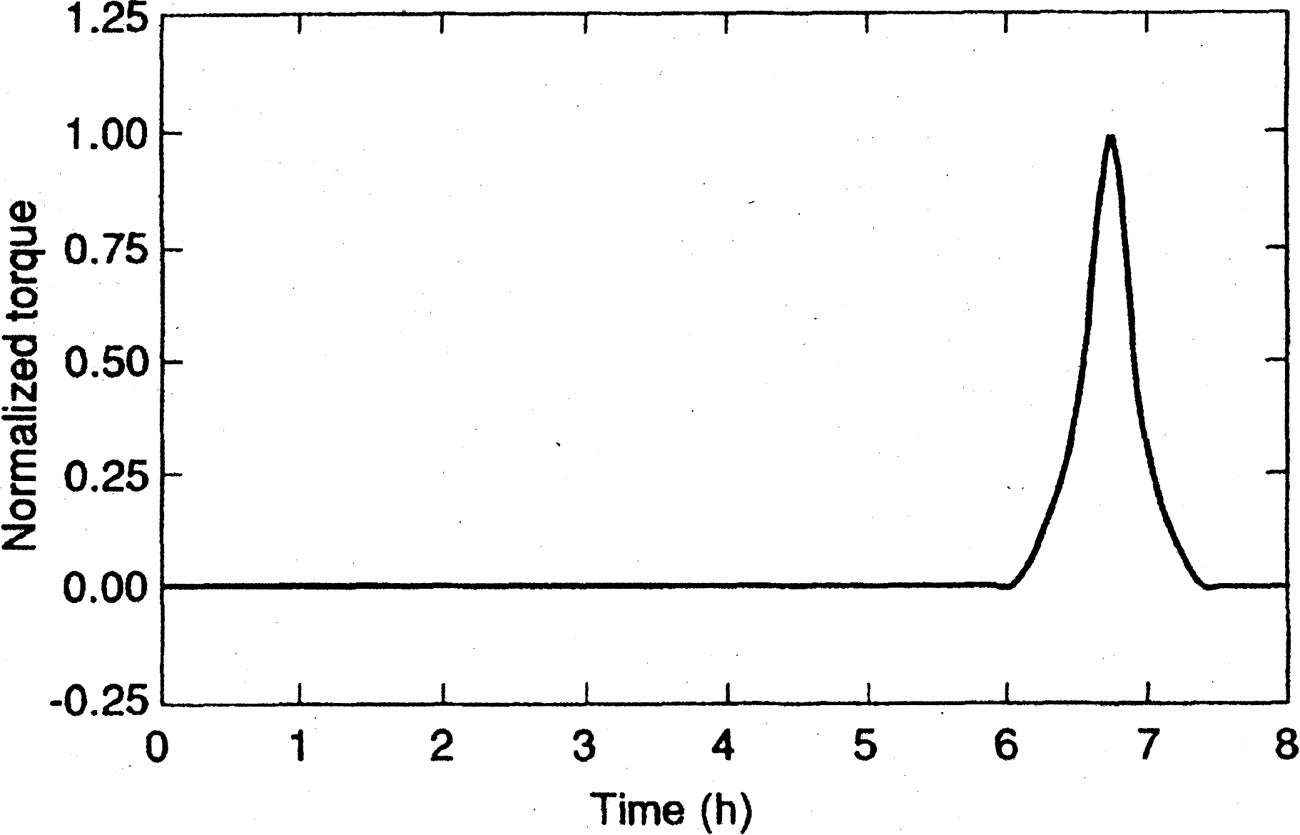
The experiment used two identical torsion balances consisting of two sapphire crystals, suspended side by side in a vacuum chamber. Shield crystals were placed in front of the sapphire disks shielding half of each disk. Thus the neutrinos would exert forces that would result in a differential rotation between the two balances, if Weber was correct. The expected differential rotation would be measured using an interferometer (Figure 15). A bar of fused silica was mounted on the top of each of the sapphire disks and positioned at an angle of 45° relative to the beams from a helium-neon laser. A rotation of the bars would increase or decrease the optical path length of the laser beams passing through them. A common rotation would have no effect on the fringe pattern, whereas a differential rotation would produce a measurable change in the pattern. The experimental apparatus was calibrated by applying a known torque to one of the torsional pendula. The estimated torque was in good agreement with the measured torque. The experimenters calculated that the apparatus would be sensitive to the neutrino flux in an interval of 20 minutes at sunrise each day. The expected result was calculated and is shown in Figure 16. A typical data run began around midnight and lasted until one hour after sunrise. A typical observed result is shown in Figure 17. “It can be seen that the signal-to-noise ratio was sufficiently high that neutrino scattering of the expected magnitude should have been apparent from a single experimental run and that none was observed” (pp. 2237–8). The data for 22 experimental runs gave an average value for f = 0.0033 ± 0.0030 where τ(t) f τ_e(t), where τ(t) was the measured torque, τ_e(t) was the expected torque, and f was a parameter obtained from a least squares at to the data. Franson and Jacobs concluded that, “The uncertainty (one standard deviation) in this estimate was obtained from the observed variation in the results of the 22 individual experimental runs and is a factor of 330 less than one would expect from total scattering ( f = 1). These results are inconsistent with the corresponding value ( f = 0.86) obtained in the earlier experiments …” (p. 2238).

The experimenters also noted that, “As mentioned previously, the expected torque τ_e(t) was calculated on the hypothesis that the shield crystals give total scattering as suggested by the earlier theoretical predictions and experimental results” (p. 2238). Without using that assumption they calculated a large-angle neutrino scattering length, l_s. Their experimental results yielded an estimated scattering length of greater than 120 cm, consistent with an infinite scattering length. The results obtained by Weber yielded a scattering length of 0.64 cm. “Thus the results obtained here are inconsistent with the earlier results regardless of whether or not it is assumed that the shield crystals scatter all of the incident neutrinos” (p. 2238).³²

Both Weber’s theory and experimental results were further tested in an experiment by the Eöt-Wash group³³ that was primarily devoted to measuring the universality of free fall (Su, Hackel et al. 1994).³⁴ The experimenters used a continuously rotating torsion balance to measure the differential accelerations of Be-Cu and Be-Al test bodies, and most importantly for our story the differential acceleration of single-crystal silicon encased in an aluminum shell and copper. The experiment was calibrated by both sudden changes in the turntable rotation speed and by placing known masses near the torsion balance to introduce known gravitational effects. Other sources of background that might mask or mimic the sought-for effect such as tilt of the apparatus, magnetic effects, thermal effects, turntable irregularities, etc. were shown to be negligible.

The data obtained with the Si-Al/Cu masses, could be used to check Weber’s results. If Weber were correct then the scattering of neutrinos from the single-crystal Si mass would change the rotation of the balance. Once again, the experimenters noted that Weber’s theoretical calculations had been refuted but that, “Here we address Weber’s claim that he has verified his theory experimentally by using neutrinos from the Sun” (p. 3634). The group stated that although their test bodies were not optimum (as compared with those used by Weber) for testing Weber’s results, “we had sufficient sensitivity to make a decisive test of Weber’s claim that solar neutrinos apply an appreciable force to single-crystal test bodies” (p. 3634). The experimenters remarked that if Weber’s results were correct then they should have observed a differential acceleration of -3.0 x 10^-9 cm/s², for the Si-Al/Cu test bodies. “Instead we saw a signal of less than -5.0 x 10^–12 cm/s² (2σ limit on Si being repelled by the Sun)” (p. 3635). This established a two-standard deviation limit of F_expt/F_Weber ≤ 1/530. “We conclude that Weber’s claim of enhanced scattering of solar neutrinos has been ruled out by direct experimental results” (p. 3635).

The combination of persuasive, negative experimental results and the theoretical arguments against the correctness of Weber’s hypothesis concerning neutrino scattering seems to have had a chilling effect on the further investigation of Weber’s idea. During the period 1994–2008, I could find only two further experimental tests of Weber’s suggestion. Both were negative.

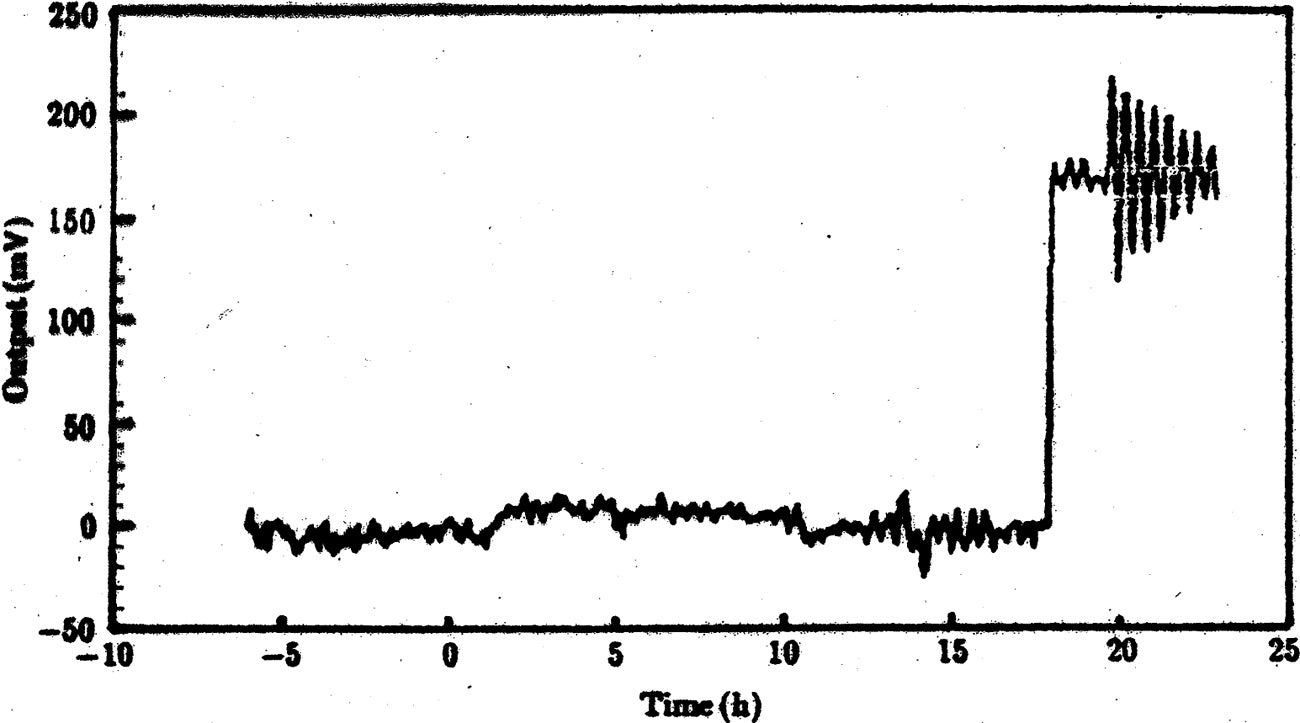
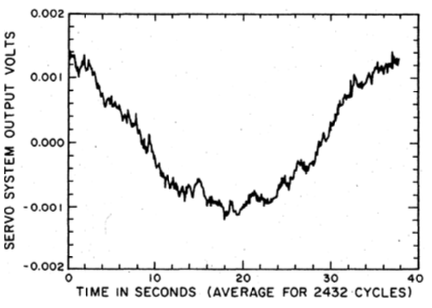
The first of these appeared in 1995 (Luo, Chen et al. 1995). The experimenters noted that Weber had not, in his original paper, given either the stability or the noise level in his torsion balance. “Reanalysing Weber’s experiments, we and that the stability or noise level of the torsion balance has not been given in his paper, this problem is very important and impossible to be neglected. If the total noise level of the torsion balance was comparative [comparable] with the effect predicted by Weber’s theory, his results, of course, would be unacceptable” (p. 137). The apparatus was quite similar to that used by Weber, containing a torsion balance with one target consisting of lead sheets and the other of a single sapphire crystal. The output for their balance is shown in Figure 18. The large jump toward the right-hand side of the figure was a calibration signal. They found that their calibration yielded a result of 1.2 x 10^-8 dynes/mV. The average noise level was 6.75 mV, yielding a noise level for the balance of 8.1 x 10^-8 dynes. They concluded, “According to the theory presented by Weber, a force of about 5 x 10^-6 dynes should be observed by our torsion balance, due to elastic scattering of solar neutrinos. Based on the ratio of 1.2 x 10^-8 dynes/mV, the predicted force 5 x 10^-6 dynes is equivalent to an output voltage of 417 mV, which was about 60 times larger than the noise level, and should be observed obviously. But, in fact, we did not and any diurnal effect larger that a force of 8.1 x 10^-8 dynes” (p. 139).

The second experiment appeared in 2006 (Feng, Yang et al. 2006). The experimenters used a torsion pendulum with four masses, two sapphire crystals and two lead rings, and measured the change in the equilibrium position of the pendulum which, if Weber was correct, would show a diurnal period. They noted the theoretical objections to Weber’s suggestion and remarked that because the Eöt-Wash group had used a different crystalline material, silicon rather than sapphire, they could not compare that result to Weber’s. “On the other hand, even though Weber’s theory is doubted, the best and valid way to test it will be a longer high precision experiment” (p. 2052). Possible temperature effects on the pendulum were carefully measured and the experimenters concluded that they were negligible. The group concluded that, “Our experiment gives a null result for the diurnal force with a noise level of 3.8 x 10^-14 N, which is 526 times smaller than the predicted value of Weber’s theory, and directly rules out Weber’s theory and the experimental result” (p. 2052).

This was the latest experimental test of Weber’s theory of neutrino scattering and of his experimental results. Over a period of slightly more than 20 years there had been only four such tests. This was a far cry from the activity occasioned by Weber’s claim that he had observed gravity waves.

Weber’s hypothesis is, however, still alive. In 2008 a group in Romania published a design for a high-sensitivity torsion balance to detect neutrinos, which they stated was currently under construction (Cruceru, Nicolescu et al. 2008). They remarked that, “One of the most intriguing proposals of the last decades in physics is represented by Weber’s idea of detecting neutrinos by coherent scattering on stiff crystals” (p. 1719). The group noted the previous theoretical criticisms of Weber, but found reasons to question all of them. They were also undeterred by the previous negative experimental tests. “Even though Weber’s theory is doubted, the only way to test it will be to go through new dedicated experiments. It is significant and necessary that new designed experiments test Weber’s theory as well as Weber’s experimental results” (p. 1725). Their new device is intended to be both highly sensitive and portable so that it can be installed in “significantly different locations.”

Discussion

The history we have presented demonstrates that “universalism” as both a norm and an ideal of science is rather complex in its application. Weber’s failure to adequately deal with the criticisms of his early work on gravity waves led to an almost total loss of his credibility with respect to that subject. Even when Weber later offered an experimental argument against the claim that he had manipulated his acceptance threshold to obtain a positive result no one in the physics community pursued that issue. It was regarded as a waste of time.

We have seen, however, that loss of credibility in one area does not necessarily lead to loss of credibility in all areas. It is not transitive. It did not apply to Weber’s unorthodox hypothesis concerning the coherent scattering of neutrinos. That suggestion, along with Weber’s experimental results supporting that claim, was investigated both theoretically and experimentally, albeit not with the same enthusiasm as his early work on gravity waves. Although that later work on neutrinos has been found wanting, it is still alive in the proposal to build a more sensitive balance, although just barely.

It is also significant that Weber’s 1984, 1985, and 1988 publications on neutrino scattering were all published in refereed journals. In fact, the 1988 paper, which included experimental results, was published after his earlier theoretical work on neutrinos had been extensively criticized. Collins cites a statement from one of the referees of that 1988 paper:

So the reason I recommended, eventually, the publication of that paper was because, although it seemed impossible, he was presenting data, he did have a calibration, and he describes his apparatus. So you can’t not publish it just because you don’t believe it can be possible. …

There were referees who said, “Don’t publish that paper,” but I said that you’d better publish it, because with my changes he’s observed all the rules. …

I was the culprit in getting that published, simply because I thought in all fairness he’s got an effect there, calibrated, and not there with a control bit of lead, and therefore it obeys all the rules of publishing, unless you just say, “Oh, it can’t be true”—a very dangerous thing to say. (Collins 2004, p. 335)

It is clear that the referee was adopting “universalism” as a guiding principle.³⁵ Although suggesting an implausible hypothesis, Weber’s paper was acceptable according to the usual criteria for publication. Neither Weber’s previous work nor the criticism of it affected the decision to recommend publication. Nor did it result in a refusal to fund his research. In his later papers Weber acknowledged financial support from the Advanced Research Projects Agency (Department of Defense), the National Science Foundation, the Defense Nuclear Agency, the Strategic Defense Initiative, and the Office of Innovative Science and Technology.³⁶

Despite Weber’s record of failure over the last 25 years of his life, his earlier achievements on the apparatus to detect gravity waves are still recognized. His original antenna, a Weber bar, along with a plaque commemorating it, is on display at the LIGO (Laser Interferometer Gravitational Wave Observatory) site in Hanford Washington. The American Astronomical Society prize for instrumentation is called the Joseph Weber Prize for Astronomical Instrumentation

This article was originally published in the Summer 2010 issue of Perspectives on Science. 

Footnotes

1. The others are: 1) Communism, in which “The scientist’s claim to ‘his’ intellectual ‘property’ is limited to that of recognition and esteem which, if the institution functions with a modicum of efficiency, is roughly commensurate with the significance of the increments brought to the common fund of knowledge (Merton 1942, p. 121). In brief, scientific knowledge belongs to the scientific community. 2) Disinterestedness, in which scientists should have no financial interest in their work. The rewards of science are through peer recognition. 3) Organized skepticism, in which the scientific investigator makes no distinction between “that which requires uncritical respect and that which can be objectively analyzed” (p. 126).

2. When the “Fifth Force,” a proposed modification of Newton’s Law of Gravity, which also violated Einstein’s Principle of Equivalence, was proposed, part of the reason for its further pursuit was the fact that it seemed to be able to explain three puzzling pieces of evidence; a composition dependent effect that showed up in a reanalysis of the Eötvös experiment, the slight difference between the value of G, the gravitational constant, when measured in the laboratory or in mines, and the tantalizing energy dependence of the CP violating parameters in Ko meson decay. In addition, the expertise of the scientists and the availability of experimental apparatus and funding may also enter into that decision. For additional discussion see Franklin (1999).

3. This is perhaps less crucial in an age when papers can be transmitted electronically or made available on the Internet.

4. For a very detailed history of this episode see Collins (2004). For a quite different view and Collins’ response see Franklin (1994) and Collins (1994). See also Levine (2004) for a participant’s account.

5. The answer to the question of whether General Relativity predicts the existence of gravity waves has changed with time. Einstein, himself, at one time thought that it didn’t, but subsequently changed his mind. For an excellent history of gravity waves see Kenneack (2007).

6. Weber’s 1970 paper, discussed below, was cited 149 times. The 1976 paper was cited 14 times, including three self-citations.

7. This is also discussed in Collins (2004, Chapters 11 and 19).

8. Weber offered no explanation of the null results obtained by his critics using his preferred analysis algorithm.

9. Garwin and Levine (1974) had shown using a computer simulation that such a procedure could generate a positive gravity wave signal. Kafka had shown the same effect using real data (Shaviv and Rosen 1975).

10. To guard against spurious signals in a single detection antenna Weber used a coincidence between two widely separated antennas to determine a gravity wave signal.

11. Drever had, in fact, looked for such pulses and found no signal (Shaviv and Rosen 1975).

12. I have no explanation for the discrepancy between Collins’s number of citations and my own except for the possibility that between 2003, when Collins did his survey, and 2008, when I did mine, the Web of Science database had improved. In any event, we both agree that these papers had no impact.

13. It is hard to imagine how another experimenter might have checked Weber’s claim. Negative results are independent of threshold. Perhaps the only way of checking Weber’s results would have been to allow another physicist to reanalyze Weber’s data.

14. One of the results that initially gave credibility to Weber’s reports was that he had observed a 24-hour periodicity in his events, which correlated with the antenna pointing to the center of the galaxy. This result later disappeared, which was just as well because gravitational waves should have shown a 12-hour periodicity. They would not be absorbed by the Earth.

15. Other papers that cited the Weber papers, but with no discussion, were Hirakawa, Tsubono, and Fujimoto (1978); Felice (1979); and Fujimoto and Hirakawa (1979).

16. One might speculate that the relatively gentle treatment that Weber’s later work received was due to the recognition of his early contributions.

17. Weber believed that such states might be achieved in polycrystalline aluminum, and single crystals of silicon and sapphire. My colleagues inform me that there is current work being done to look for quantum effects in macroscopic materials, although the systems being studied are far smaller than those Weber had suggested.

18. Virtually no one in the physics community agreed.

19. It is difficult to know whether this was because Weber stopped submitting papers to refereed journals or whether he submitted papers that were rejected.

20. This was in line with Weber’s previous attempts to demonstrate increased sensitivity for his gravity wave detectors.

21. For a macroscopic object the number of scatterers would be of the order of Avogadro’s number, 6 x 10²³.

22. This was the size of the detector used by Ray Davis and his collaborators to detect solar neutrinos.

23. These details would appear in Weber (1988).

24. Butler noted that Bertsch and Austin had used a similar argument to disallow Weber’s result.

25. For a wavelength of order 1 cm the energy of the neutrinos would be approximately 10^-4 eV, whereas the energy of the electrons used in Weber’s experiments had far shorter wavelengths, and energies in the keV and MeV range.

26. The lattice spacing would be of order 10^-8 cm, in comparison to the 1 cm size of a crystal.

27. Casella had a preprint of Butler’s paper.


28. They cited Ho, Bertsch and Austin, and Casella.

29. For details see Franklin (2001).

30. They also noted that the experiments of Dicke and Braginsky, which were used to establish the equality of gravitational and inertial mass, would be sensitive to a neutrino wind from the sun if Weber were correct.

31. In this case Weber’s theory and results acted as an “enabling theory,” which allowed the design of the Franson-Jacobs experiment. I note than an “enabling theory” need not be correct. For details see Franklin (1995).

32. Franson and Jacobs thanked Joseph Weber for a discussion of his earlier experiments.

33. The group adopted the whimsical nickname Eöt-Wash because of their previous work of the Fifth Force in which they performed a modern version of the original Eötvös experiment, which had established the equality of inertial and gravitational mass. (For details see Franklin 1993).

34. Eric Adelberger, one if the leaders of the group, “found Weber’s experiment to be completely unconvincing and realized that with a modest effort we could test his claim” (private communication).

35. One should not extrapolate from the comments of one referee. Clearly other referees argued against publication but it is difficult to know whether this was because they believed Weber’s work was wrong or because of his reputation, or some combination of the two. Nevertheless the fact that the 1984, 1985, and 1988 papers were published argues for an overall universalism in the publication process.

36. For details of Weber’s subsequent publications and funding see Collins (2004, Chapters 19–21).

References

Aharonov, Y., F.T. Avignone, A. Casher, et al. 1987. “Constraints on
Anomalous Scattering of Neutrinos from Crystals.” Physical Review Letters 58: 1173–1175.

Bertsch, G.F. and S.M. Austin.1986. “Comment on “Method of observation of neutrinos and antineutrinos.” Physical Review C 34: 361. 

Boughn, S.P., W.M. Fairbank, R.P. Giffard, et al. 1982. “Observations with a Low-Temperature Resonant Mass Gravitational Radiation Detector.” Astrophysical Journal 261: L19–L22. 

Brown, B.L., A.P. Mills and J.A. Tydon 1982. “Results of a 440-day search for gravitational radiation.” Physical Review D 26: 1209–1218. 

Butler, M.N. 1987. “Comment on “Method of observation of neutrinos and antineutrinos.” Physics Review C(1164–1165). 

Casella, R.C. 1986. “On the Theory of Coherent Neutrino Scattering from Crystals.” Il Nuovo Cimento 94A: 42–50.
Collins, H. 1994. “A Strong Confirmation of the Experimenters’ Regress.” Studies in History and Philosophy of Modern Physics 25(3): 493–503. 

Collins, H. 2004. Gravity’s Shadow. Chicago: University of Chicago Press. 

Cruceru, I.,G. Nicolescu and O.G. Duliu. 2008. “A Dedicated Torsion Balance to Detect Neutrinos by Coherent Scattering on High Debye
Temperature Monocrystals.” International Journal of Modern Physics A
23: 1719–1728. 

Dodonov, V V., V.I. Manko and V.N. Rudenko. 1982. “The Efficiency of
a Gravitational Detector with Interference of Quantum States.” JETP
Letters 36: 63–66.

Dodonov, V.V., V.I. Manko and V.N. Rudenko. 1983. “Quantum Integrals of Motion and Gravity-Wave Experiment Measurements in Pure
Quantum Sates.” Foundations of Physics 13: 607–628.

Drever, R.W.P., J. Hough, R. Bland, et al. 1973. “Search for Short Bursts
of Gravitational Radiation.” Nature 246: 340–344.

Felice, F.D. 1979. “Effects of a Graviational Wave on Relativistic Particles.” Journal of Physics A 12: 1223–1228.

Feng, G., S. Yang, L. Tu, et al. 2006. “Improvement of Test of Solar Neutrino Coherent Scattering with Torsion Pendulum.” Chinese Physics Letters 23: 2052–2055.

Ferrari, V., G. Pizzella, M. Lee, et al. 1982. “Search for correlations Between the University of Maryland and the University of Rome
gravitational radiation antennas.” Physical Review D 25: 2471–2486.

Franklin, A. 1993. The Rise and Fall of the Fifth Force: Discovery, Pursuit,
and Justiacation in Modern Physics. New York: American Institute of
Physics.

Franklin, A. 1994. “How to Avoid the Experimenters’ Regress.” Studies in
History and Philosophy of Modern Physics 25: 97–121.

Franklin, A. 1995. “Laws and Experiment.” Pp. 191–207 in Laws of Nature. Edited by F. Weinert. Berlin: De Gruyter.

Franklin, A. 1999. “Instrumental Loyalty and the Recycling of Expertise.”
Pp. 97–131 in Can That Be Right? Essays on Experiment, Evidence, and
Science. Edited by A. Franklin. Dordrecht: Kluwer Academic Publishers. 

Franklin, A. 2001. Are There Really Neutrinos? An Evidential History. Cambridge, MA: Perseus Books.

Franson, J.D. and B.C. Jaqcobs 1992. “Null Result for Enhanced Neutrino Scattering in Crystals.” Physical Review A 46: 2235–2239.

Fujimoto, M.K. and H. Hirakawa. 1979. “Theory of the Detection of
Continuous Gravitational Radiation.” Journal of the Physical Society of
Japan 46: 703–706.

Garwin, R.L. 1974. “Detection of Gravity Waves Challenged.” Physics Today 27(12): 9–11.

Giffard, R.P. 1976. “Ultimate sensitivity limit of a gravitational wave antenna using a linear motion detector.” Physical Review D 14: 2478–
2486.

Hirakawa, H., K. Tsubono and M.K. Fujimoto. 1978. “Search for Gravitational Radiation from the Crab Pulsar.” Physical Review D 17: 1919–
1923.

Ho, T.H. 1986. “Comments on the “Method for Observation of Neutrinos and Antineutrinos”.” Physics Letters 168B: 295.

Jensen, O.G. 1979. “Seismic Detection of Gravitational Radiation.” Reviews of Geophysics and Space Physics 17: 2057–2069.

Kafka, P. and L. Schnupp. 1978. “Final Result of the Munich-Frascati
Gravitational Radiation Experiment.” Astronomy and Astrophysics 70:
97–103.

Kenneack, D. 2007. Traveling at the speed of thought: Einstein and the quest for
gravitional waves. Princeton: Princeton University Press.

Lee, M., D. Gretz, S. Stepple, et al. 1976. “Gravitational-Radiation-Detector Observations in 1973 and 1974.” Physical Review D 14: 893–
906. 

Levine, J.L. 2004. “Early Gravity-Wave Detection Experiments, 1960–1975.” Physics in Perspective 6: 42–75.

Lipkin, H.J. 1987. “N Dependence of Coherent Radiation from Crystals.”
Physical Review Letters 58: 1176–79.

Luo, J., X. Chen, J. Li, et al. 1995. “Test of Abnormally Large Coherent
Scattering Cross section Using Solar-Neutrino.” Chinese Physics Letters
12: 136–139.

Merton, R. 1942. “A Note on Science and Democracy.” Journal of Legal
and Political Sociology 1: 115–126.

Shaviv, G. and J. Rosen, Eds. 1975. General Relativity and Gravitation: Proceedings of the Seventh International Conference (GR7), Tel-Aviv University,
June 23–28, 1974. New York: John Wiley.

Smith, P.F. 1987. “Comments on a reported Measurement of an Abnormally Large Coherent Neutrino Cross Section.” Physics Letters B 183:
107–109. 

Su, Y., B.R. Hackel, E.G. Adelberger, et al. 1994. “New Tests of the
Universality of Free Fall.” Physical Review D 50: 3614–3636.

Tyson, J.A. and R.P. Giffard. 1978. “Gravitational-Wave Astronomy.”
Annual Reviews of Astronomy and Astrophysics 16: 521–554.

Walls, D.F. and P. Zoller. 1981. “Enhanced Sensitivity of a Gravitational
Wave Detector.” Physics Letters 85A: 118–120.

Weber, J. 1969. “Evidence for Discovery of Gravitational Radiation.”
Physical Review Letters 22: 1320–1324.

Weber, J. 1970. “Anistropy and Polarization in the Gravitational-Radiation Experiments.” Physical Review Letters 25: 180–184.

Weber, J. 1977. “Gravitational Radiation detector observations in 1973
and 1974.” Nature 266: 243.

Weber, J. 1981. “New Method for Increase of Interaction of Gravitational
Radiation with an Antenna.” Physics Letters 81A: 542–544.

Weber, J. 1984. “Gravitons, Neutrinos, and Antineutrinos.” Foundations of
Physics 14: 1185–1209.

Weber, J. 1985. “Method for observation of neutrinos and antineutrinos.”
Physics Review C 31(1468–75).

Weber, J. 1988. “Apparent Observation of abnormally large coherent scat-
tering cross sections using keV and MeV range antineutrinos, and solar
neutrinos.” Physical Review D 38: 32–39.

Weber, J., M. Lee, D.J. Gretz, et al. 1973. “New Gravitational Radiation
Experiments.” Physical Review Letters 31: 779–783. 

• Allan Franklin

  Posted on March 2, 2017

  Allan Franklin is a professor at the University of Colorado.

Get the Nautilus newsletter

Cutting-edge science, unraveled by the very brightest living thinkers.

Newsletter Signup – In Page Mobile

Email: *

Captcha

If you are human, leave this field blank.

Sign up for free