The water is always running in Björn Hof’s laboratory.
Like a Zen water fountain, it gently flows over the top of a reservoir into a tube, and from there into a glass pipe 15 meters long, but thinner than a glass thermometer. To keep the flow as smooth and serene as possible, Hof, of the Institute of Science and Technology Austria in Klosterneuberg, controls conditions such as temperature and sterility of the tube as meticulously as might a biologist trying to breed a particular strain of bacteria.
And in a way, Hof is trying to breed a strain of reproducing creatures, just not living ones. Into the Zen-like perfection, he occasionally adds a pinprick of confusion: a tiny amount of water injected through the side of the tube. As each “puff” of swirling water travels down the pipe, it may divide into two puffs like a self-replicating bacterium, or it may just as suddenly die out.
The dynamics of this population of puffs, Hof believes, holds the key to a problem that has vexed physicists for over a century: How does turbulence get started, and what is it, anyway?
It’s been more than 130 years since an English engineer named Osborne Reynolds launched the study of turbulence with an experiment not so different from Hof’s. To make turbulence visible, Reynolds injected dye into water flowing through a glass pipe. When the water flowed slowly, he found that the dye traced a straight line that did not spread out—what researchers call smooth, “laminar” flow. At a faster flow rate, the dye would meander a bit. But when the water flowed just a bit faster still, it abruptly became turbulent: The dye would spread out in wild curlicues, which quickly filled the entire width of the pipe.
The critical Reynolds number for flow through a pipe was quite possibly the worst-known constant of nature.
Water flowing through a pipe is perhaps the least complicated regime in which to study turbulence. But, amazingly, researchers have still not fully explained Reynolds’ observations. “People ask me, ‘how can this not be solved, after all these years?’ ” says Tom Mullin of the University of Manchester, the same university where Osborne did his experiments.
It’s not that the stakes are low. A thorough explication of turbulence in pipes could help illuminate the transition to turbulence in a wide range of settings. Understanding how to minimize turbulence in air and fluids could ultimately help engineers pump oil through long pipelines more efficiently and build cars that generate less wind resistance. It could also allow them to harness turbulence more effectively in the settings in which it is helpful, as when vortices near an airplane wing pull a smooth layer of air toward the wing and allow the plane to come in for a slower and gentler landing.
Within the last 10 years, the obstinate problem of how turbulence gets started in a pipe has finally begun yielding its secrets. In 2004, Bruno Eckhardt of the University of Marburg and Rich Kerswell of the University of Bristol discovered, at least in theory, an elusive third state between laminar flow and turbulence called a traveling wave. This kind of wave manifests itself in experiments as the puffs Hof creates in his long glass tube. In 2011 Hof, along with five collaborators, used these puffs to unravel the story of how turbulence begins. Puffs, they propose—while not turbulent themselves—are in some sense the atoms of turbulence.
“They put the final piece into the puzzle,” Eckhardt says. “You can debate the details and the numbers, but we have a clear understanding of what we should look at now, so that we can apply the same methods to other systems.”
Fluid Zip Code
Fluid flow (including air flow, because air is a kind of fluid) is governed by a set of rules called the Navier-Stokes equations, which were known even before Osborne Reynolds did his experiments. In theory, understanding fluid flow in a pipe is purely a matter of mathematics: Plug in the pipe’s dimensions, and the velocity and pressure of the water at the inlet, solve the Navier-Stokes equations, and you’re finished.
But that’s easier said than done. The Navier-Stokes equations contain what mathematicians call a nonlinearity, a sort of feedback loop by which a vortex can suck in energy from the rest of the flow to become stronger and stronger. As scientists discovered in the 1960s and 1970s, nonlinearity is a breeding ground for chaos. Even the slightest alteration to the flow, one too small even to be detected, can completely alter its subsequent behavior. That is why we still have trouble predicting the weather beyond five days in the future.
Pipe flow is one of the few cases in which the Navier-Stokes equations do have a simple solution: laminar flow. And in theory, this solution is stable, like a well-balanced canoe. According to the equations, laminar flow will never tip over, so to speak, and capsize into some other state. But in practice, it always does, if the water is moving fast enough. When you turn the spigot on full blast, you don’t see a smooth, crystal clear stream. You see a churning, complicated mess. This makes pipe flow an important test case for turbulence: What is the mechanism for the “canoe” tipping over, when it seems to start out perfectly balanced?
Puffs are not just decaying—they are also reproducing.
To add to the difficulty of the problem, scientists have not even agreed on how to define turbulence to the present day. Depending on whom you ask, turbulence means rapid mixing, vortex stretching, an energy cascade from larger to smaller vortices, or sensitive dependence on initial conditions.
Yet researchers do have a sort of atlas for studying turbulence: a simple ratio, discovered by Reynolds, which sums up the physical state of a moving fluid. This “Reynolds number,” which combines the fluid’s speed and viscosity, is a great equalizer that permits scientists to describe all (or almost all) fluids in the same way. Thus they can experiment on a small wind tunnel and scale the results up to an airplane, or experiment on water to draw conclusions about the flow of oil.
For fluid researchers, a Reynolds number is like a zip code. Below 1,000, where the fluid is viscous or slow, you are in the realm of laminar flow. Between 1,000 and about 2,000, where the fluid is flowing faster, you can introduce disorder but it will die out. And somewhere right around 2,000, Reynolds observed, the fluid undergoes a transition: It becomes a hospitable environment for turbulence. Between 2,000 and 4,000, the proportion of turbulent fluid in the pipe increases from near zero to near 100 percent.
Until now, fluid researchers have struggled to understand just what is going on at the transition to turbulence, and even to pin down at exactly which Reynolds number this transition occurs. In 2009, Eckhardt observed that different versions of Wikipedia gave different values for this critical Reynolds number: In English, French, and Swedish, it was said to be 2,300; in German, 2,320; in Portuguese, between 2,000 and 3,000; and in Spanish, between 2,000 and 4,000.
In any other field of physics, such uncertainty would be a scandal. The critical Reynolds number for flow through a pipe was quite possibly the worst-known constant of nature.

Life and Death
Now, Hof’s experiment has finally brought some clarity to the question. The experiment is rooted in the 2003 discovery by Eckhardt and Kerswell of the first bona fide mathematical solutions, aside from laminar flow, to the Navier-Stokes equations in a pipe. (Their work built on a previous discovery by Fabian Waleffe of the University of Wisconsin of similar structures in a flow bounded by two flat plates.) These solutions, which begin to appear between Reynolds numbers 773 and 2,000, are neither laminar nor turbulent. Instead, they feature pairs of counter-rotating vortices that drift downstream without either dissipating or intensifying.
These vortices, called traveling waves, are purely a computer construct. They are unstable, so you can no more produce one in the laboratory than you can balance a needle on its point. However, it is possible to create a sort of traveling wave wannabe—called a puff—that has a similar structure to a traveling wave but which persists long enough in the laboratory to be measured.
Although a puff swirls around, it does not constitute full-on turbulence—it’s more like a seed of turbulence. Unlike turbulence, a puff is limited in space, and doesn’t spread out to the entire pipe. Perhaps even more importantly, it is limited in time. A puff will be floating down the pipe, showing no signs of ill health, when suddenly—poof!—it’s gone, and the water goes back to laminar flow.
Eckhardt and his team were the first people to argue that all puffs are transient, even above the critical Reynolds number. Before his 2004 paper, researchers had assumed that puffs become immortal above the critical number, and that this permanence explained the transition to turbulence. Hof’s experiment has proved that Eckhardt was right: Puffs continue to have a finite life span even above Reynolds number 2,000. But this creates a paradox. If puffs are transient, how can they give rise to steady-state turbulence? “That was a hotly debated topic for four or five years,” says Dwight Barkley of the University of Warwick, who collaborated on Hof’s experiment.
The key, Barkley and Hof realized, lay in understanding what happened to the puffs before they died. Around 1975, Israel Wygnanski of the University of Arizona had noticed that one puff sometimes spontaneously divides in two. So puffs are not just decaying—they are also reproducing.
Like radioactive nuclei, puffs have a measurable decay rate. Nobody can predict when an individual puff will vanish, but if you have a large enough collection of puffs, you can say exactly what percentage will vanish in a given time. Likewise, the appearance of individual new puffs is unpredictable, but taken as a whole, the population of puffs reproduces at a predictable rate. Hof, Barkley and their collaborators—Kerstin and Marc Avila of the University of Erlangen, David Moxey of Imperial College London, and Alberto de Lozar of the Max Planck Institute in Göttingen—found that as the Reynolds number increases, the birth rate of puffs rises while the death rate goes down.
Even Thomas Malthus could have predicted what happens next. As soon as the birth rate exceeds the death rate, turbulence will spread. It’s as if the pipe becomes overpopulated with puffs. If the birth rate is less than the death rate, turbulence will decay. And the place where the birth and death rates are exactly equal is the critical Reynolds number, where the transition to turbulence occurs.
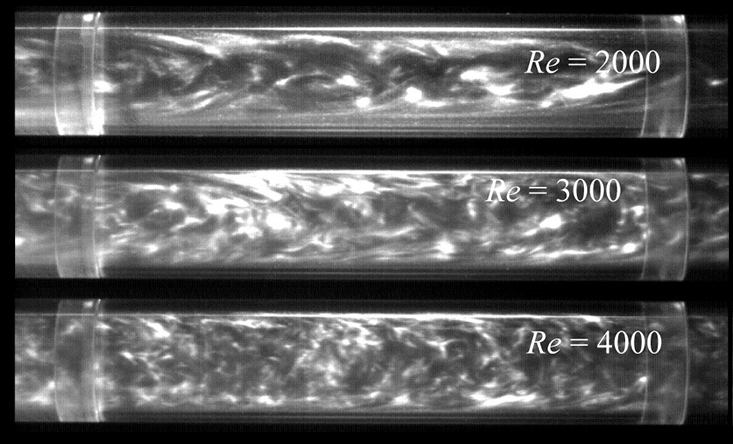
It’s a simple, beautiful idea. But pinning the critical Reynolds number down experimentally was not easy. As the flow approaches the critical Reynolds number, the half-life of a puff grows dramatically. In a pipe whose diameter is one centimeter, at Reynolds number 1,800 you would expect half the puffs to die out after flowing along for just one meter. But if you crank it up to 2,000, you would need a pipe more than 60 miles long to see half the puffs die out. Building so long a pipe was out of the question, and computer simulations wouldn’t do the trick either, because the best supercomputers today are about a thousand times too slow for the job.
There was, nevertheless, a way forward. As Barkley points out, “we know the half-life of many things. For carbon-14 [whose half-life is 5,730 years], we know it not by looking at a single atom for 5,000 years, but by watching a whole lot of atoms.” Likewise, you can estimate the birth and death rates for puffs by watching a large collection of them in action. Hof built an automatic puff generator and sent about a million puffs through his 15-meter pipe, enough to get a few hundred puffs to decay or reproduce.
The researchers found that the birth and death rates equalized at a Reynolds number of 2,040. It was a vindication for Reynolds, whose experiment in 1883 came closer to the right answer than did most of the Wikipedia entries from 2009.
Beyond Pipes
Barkley, Hof, and others are now pushing beyond Reynolds number 2,040 to figure out what happens to turbulent flows after they have reached the transition zone. Counter to Reynolds’ observations, the flow does not become entirely turbulent right away: Turbulent zones are interspersed with smooth regions. If two or three puffs in a row die without reproducing, which can easily happen if the Reynolds number is only a little greater than 2,040, then you will get a long section of laminar flow.
Meanwhile, Eckhardt and Waleffe are interested in extending the idea of puffs to other turbulent structures, such as the flow of air over an airplane wing. The Reynolds number in this application is not constant, but starts at zero on the leading edge of the wing and grows to 10 million or more at the trailing edge. Intelligently designed structures could have a significant impact when placed on the parts of the wing where the transition to turbulence occurs. Many airplanes already have little vertical fins on the wings, called vortex generators, designed to strategically increase turbulence when a plane is taking off or landing. But, Waleffe points out, these structures were not designed from an understanding of the physics. “They were discovered very much by trial and error, shooting in the dark,” he says. Aeronautical engineers do not usually pay attention to the study of fluid flow in pipes, he notes, but if puffs could be shown to play an important role in other fluid-flow problems, maybe they should.
The most important thing about the puffs may not be any particular application but the clarity they provide. In spite of (or perhaps because of) all the different definitions of turbulence, up to now there has been no clear way to specify when it begins. The work of Hof’s group suggests a clear definition: Turbulence emerges when the birth rate of puffs exceeds the death rate, and the puffs are able to colonize the fluid.
Eckhardt believes that this definition, quantifiable and precise, could serve as a diagnostic for turbulence in other applications as well, not just pipe flow. It will allow researchers “to gauge anything they want to do to the flow,” he suggests, whether they are adding fins to an airplane wing to encourage turbulence or adding polymers to oil to discourage it. “It’s always a good thing to get the basics straight.”
Dana Mackenzie is a freelance mathematics and science writer based in Santa Cruz, California. His most recent book is The Universe in Zero Words: The Story of Mathematics as Told Through Equations.
This article was originally published in our “Turbulence” issue in July, 2014.