Ladies and gentlemen, the captain has turned on the seat belt sign. Please return to your seats and ensure your seat belts are fastened.” So goes the familiar prelude to the disconcerting mid-air jostling most people associate with the term turbulence. But turbulence is far more common and far more beautiful than this bumpy ride might lead one to think.
In fluid dynamics, the science of how liquids and gases move, turbulence is a state of being. Any flow may be, or may become, turbulent. In fact, at the human scale, most flows are turbulent. The flickering flames of a campfire, the white-capped froth of river rapids, and the swirling eddies of milk poured into coffee are all examples of everyday turbulence.
Turbulent flows often appear random or chaotic at a glance. Who could, for example, predict the exact way a drop of ink will curl and split when dropped in a glass of water? The complexity of turbulence is part of its beauty. Spend an hour watching the clouds roll overhead and no two of them will be the same. The complexity and dynamic motion of turbulence make these flows fascinating, even mesmerizing, to watch. Humans are a pattern-seeking species. We like to seek order in apparent chaos, and this, perhaps, is what makes turbulence such a captivating subject for scientists and artists alike. (See “The Scientific Problem That Must Be Experienced,” about the different insights that scientists and artists bring to turbulence.)

But while that unpredictability often reads as beauty to the spectator, it is an intense challenge to the scientist. Turbulence was, and is, one of the great unsolved mysteries of classical physics, and many of the most brilliant scientists, engineers, and mathematicians of the past two centuries have tried to unravel the patterns inside it. In some sense, the problem of turbulence was solved long ago. The equations that describe fluid motion, the Navier-Stokes equations, have been known for more than a century and half. They are essentially Newton’s second law of motion (which itself was laid out a good two hundred years before) written for a fluid, describing flows both turbulent and non-turbulent. But they are not what anyone would call simple equations; when applied to any real-world scenario, they are in fact horribly complicated. Not even the largest, fastest supercomputers in the world can fully solve these equations for a practical problem, such as airflow around a car or combustion inside an engine. (See “To Predict Turbulence, Just Count the Puffs,” about one researcher’s quest to describe a simplified version of the phenomenon: the turbulence inside pipes.)
Though we still lack a simple way to exactly predict turbulence, the many research hours poured into the question have yielded some results. In his autobiography, aerodynamicist Theodore von Kármán recounted his efforts in 1930 to predict the velocity of turbulent flows near a wall. Engrossed in the attempt to find a simple equation that would describe their experimental observations, von Kármán and his assistant worked one night until time to catch the final streetcar. The two continued their calculations on the street corner, going so far as to write their equations on the side of the waiting streetcar. Finally the conductor would wait no longer. Von Kármán’s assistant managed to scramble aboard and spent the trip home jumping off at every stop to write down more of the mathematics. Their “law of the wall” was originally meant to mathematically describe turbulent flow past a flat plate, but subsequent researchers found the equation applicable in many more situations. Water near river bottoms, flow through a pipe, and wind speed in the lowest part of the atmosphere all exhibit a form of the law. Yet the pattern they found is far from able to describe turbulence as a whole.
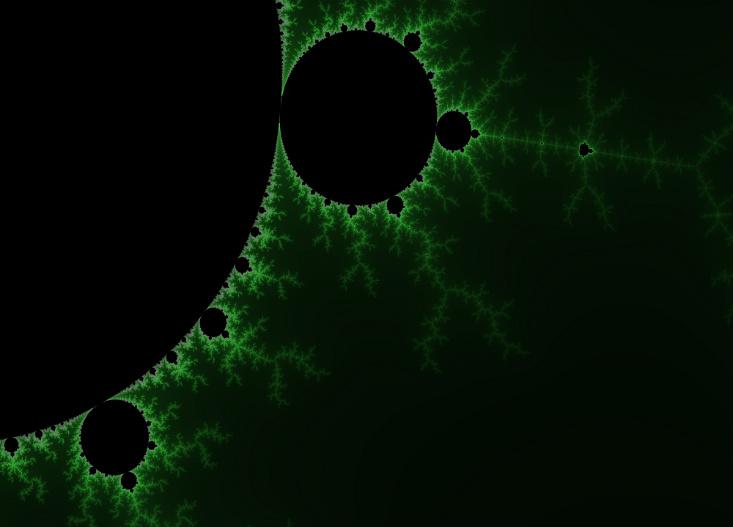
One key to getting a better handle on turbulence is understanding its propensity to repeat on different size scales. A volcanic plume may be kilometers long, with eddies hundreds of meters across, that slowly spin and turn over on themselves. At the same time, many smaller eddies, all the way down to the scale of centimeters or millimeters, are also moving and swirling and mixing—much like the drop of milk in your coffee. A powerful way of looking at these systems came in the 20th century from mathematician Benoit Mandelbrot. While working at IBM, Mandelbrot began exploring what he would come to call fractals: mathematical sets that contain repeating geometric patterns at every scale. Zoom in on the Mandelbrot set and the same shapes repeat themselves over and over infinitely. This characteristic is known as self-similarity, and it occurs, at least above the molecular scale, many times in nature. Fractal patterns have been found in coastlines, lightning bolts, vegetables, and even the timing of heartbeats.
Self-similarity also makes fractals an appealing tool for those looking to find a pattern in the many scales of turbulence. In fact, Mandelbrot himself pointed out that the concept of self-similarity was created to describe turbulence decades before his work with fractals. Today, among other applications, fractals are used to describe the geometry of turbulence, like the complicated boundary of a cloud or that volcanic plume. (See “In the Mind of the Fractal King,” Nautilus’ posthumous “interview” with Mandelbrot.)
In situations when scientists can’t use a simplified approach to predicting turbulence, they instead solve approximations of the Navier-Stokes equations, replacing the problematic terms with simpler models and approximations. Practical problems become computationally tractable, but the results may not capture reality correctly. This is one of the great challenges in weather forecasting, where modeling is necessary but may or may not predict a given day’s weather accurately.
In the end, whether we are meteorologists trying to predict a storm’s path or airline passengers intent on keeping coffee out of our electronics, we are all searching for patterns in the turbulence. There is a rich complexity to the subject, a beauty and depth sufficient to fascinate both the researcher and the layman. Perhaps one day someone will find the right pattern—the perfect pattern—to reduce turbulence’s complexity. Fortunately, spending an afternoon watching the surf or a night appreciating a campfire will remain as entrancing regardless.
Nicole Sharp has a Ph.D. in aerospace engineering and writes regularly about fluid dynamics at her blog FYFD.



























