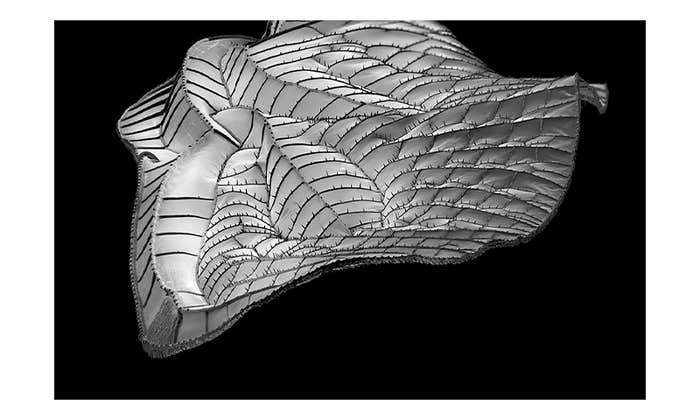
A rose is a rose is a … geometrical marvel? Scientists have long wondered how rose petals achieve their iconic shape. It turns out that a unique type of stress forges the rose’s graceful curves and curls.
Lots of plants owe their form to the push and pull of physical forces. Their tissues often grow unevenly: For instance, the edge of a leaf may grow more quickly than its interior, so the leaf takes on a ruffled shape to account for this mismatch. This is driven by a phenomenon called Gauss incompatibility, which explains the waves commonly found on the edges of petals, leaves, and seed pods.
But rose petals are different, according to recent findings published in Science. The researchers suggest, instead, that the shape arises from another type of rule-breaking. The Mainardi-Codazzi-Peterson condition states that curves evolve smoothly and consistently across a surface. Rose petals violate this condition, sparking geometric frustration and accumulating concentrated stress at petal cusps during growth. This incompatibility forges the sudden sharp edges on the tips of the petals.
The team tested this theory by cutting strips from rose petals in multiple directions, creating faux plastic petals to mimic the growth process, and running computer models. Their findings revealed that the immense stress fixed on petal cusps affects how the surrounding tissue grows. As the petal matures, smooth curves morph into polygonal shapes with sharp, multiplying cusps. The team also suggests that understanding the forces at play in developing rose petals could inspire designs for self-morphing materials in robotics and spaceships. ![]()
Lead image by Dr. Yafei Zhang